This is my search for Chodesh: The New Moon worship day; just what and where is it?

Further, whether anyone wants to keep the day or not, this investigation brings out a lot of fascinating points that anyone studying the Bible will find extremely interesting:

• how is the Bible month constructed?
• how long is it?
• when does the Bible month start and end?
• there are currently two lunar months. Why are they different? [Actually there are more but mainly two that are reasonably well known]
• and a lot of fascinating information about the moon!

I started on this search with the only understanding of chodesh or the Bible new moon day basically just the new moon mentioned on my wall calendar. Anyone reading this should consider this as a reasonable starting point in their mind.

The conclusions that I have drawn and the day I have constructed are not the same as the traditional Jewish new moon day. The Bible reasons for this are given. I may be wrong but this works for me. The working solution is very simple and based on the fact that the phases of the Moon appear the same everywhere on the Earth because of the distance of the moon from the Earth. Though not the intent, the numerical solution could also be worked just as easily for the Jewish calendar though not really necessary since these calendars are readily available. Note this is nothing fancy—it's just a number to subtract from the astronomical date to get the correct day—approximately.

The numerical solution obtained was tested with the Jewish calendar for 2011 and in every case succeeded in landing on a Rosh Chodesh date. Some months the Jewish calendar listed 2 Rosh Chodesh days. Every time this happened the numerical solution was successful in landing on one of them. Further, using the Jewish calendar it was possible to make a small correction in the numerical solution if one wanted to use it to obtain Jewish new moon days.

So toss away any preconceived ideas you may have of this day and come on a curious walk as we wander about trying to figure out just what and where chodesh is.

Note: chodesh is the Hebrew word that is translated mostly as month, but sometimes as new moon depending on the context in the verse.

The search for chodesh

Very early in my Christian walk I came across Isaiah 66:22,23:

For as the new heavens and the new earth, which I will make, shall remain before me, saith the LORD, so shall your seed and your name remain.
And it shall come to pass, that from one new moon to another, and from one sabbath to another, shall all flesh come to worship before me, saith the LORD.

This was certainly not a problem. I believed Isaiah was here clearly stating that there would be a weekly worship day of rest, a sabbath, which will be kept by God's people for eternity. There was also mention of another worship day, the new-moon, whatever that entailed. It was not very clear and I have not heard much about it if anything at church. For all the years I have been a Christian I have never heard a sermon about this extra day of worship.

The years rolled by. As a Christian I referred to these verses many times but as usual left the new-moon part in the too-hard-basket. Probably like most other Christians who were travelling this road.

Late in 2010 this all changed. I was studying some verses in the Old Testament and realised that they definitely had a worship connection with the new moon. The new moon itself was not mentioned in the verses but to me it was very clear that the worship being outlined clearly was linked to the new moon worship day. And the worship I was looking at had absolutely nothing to do with the Law of Moses containing sacrifices and ordinances. By new moon here, I mean specifically a day of worship as outlined in Isaiah:

“one new moon to another, ... shall all flesh come to worship...”

From this point onwards I decided that this new moon worship day needed another look.

So I decided to search for this worship day. Where would you start? Well the most obvious place to start looking for a new-moon worship day is of course, the new-moon.

The first place to look was on the internet for sites that might list moon phase dates. This was successful and the first chart I obtained was as follows:


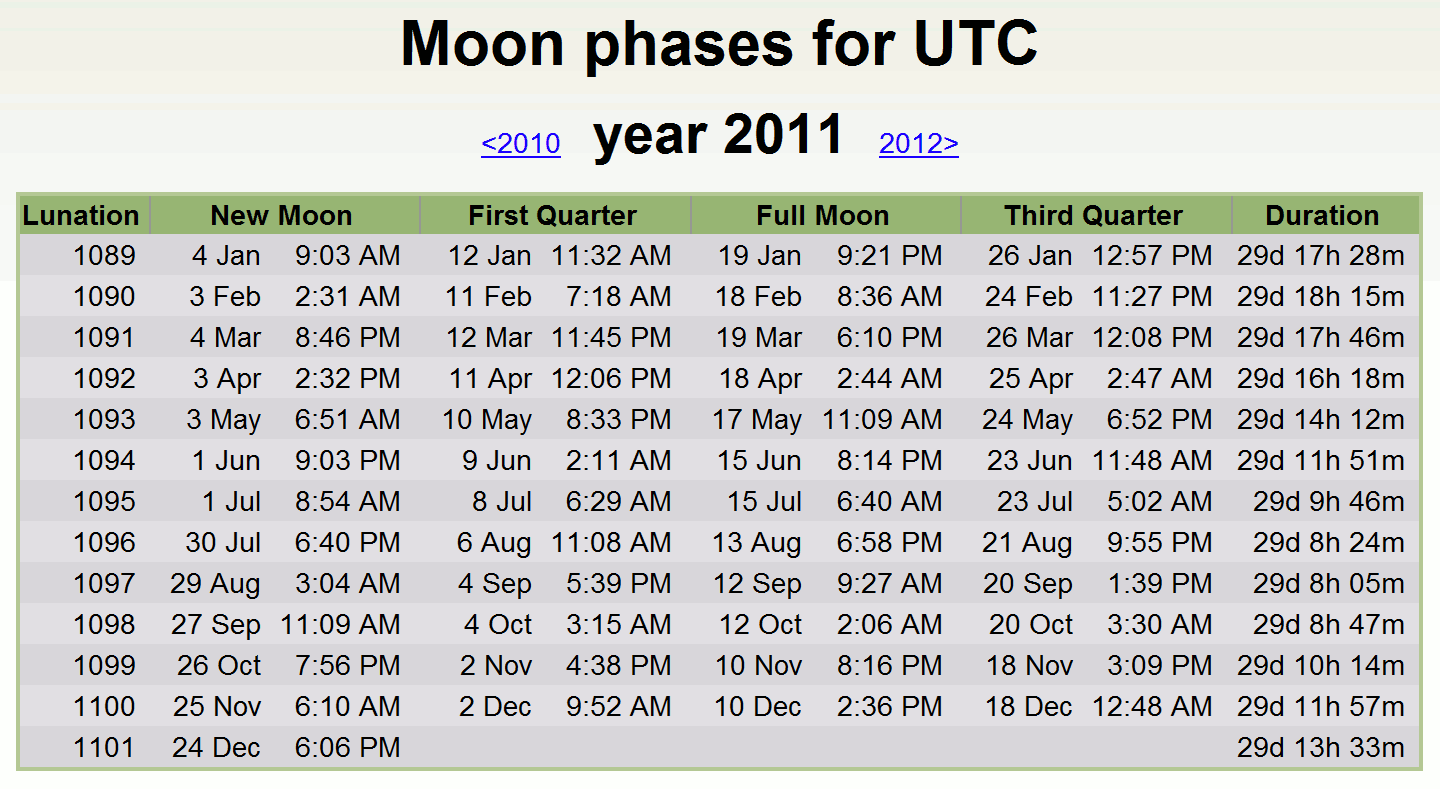
From http://www.timeanddate.com/calendar/moonphases.html

These dates were for UTC which I assumed was a certain time or location so checked where I could adjust this on the page. Setting the location for my approximate time location I then obtained the chart:


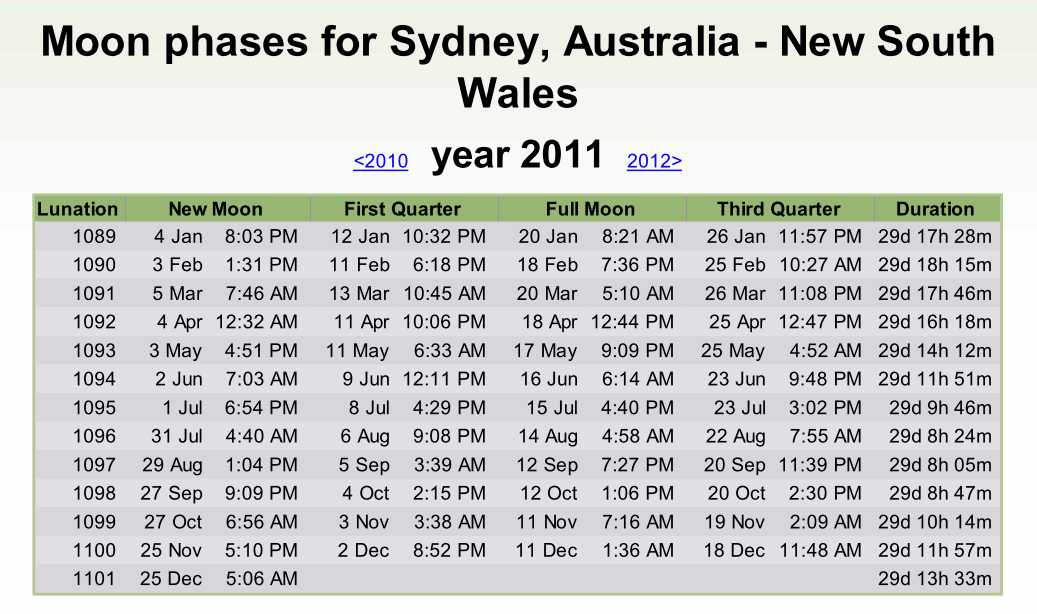
From http://www.timeanddate.com/calendar/moonphases.html

From here, it begins to get very interesting.
The next step was to determine the correct length of time for this worship.
The comment by Isaiah infers possibly a day. But how long does a phase of the moon last? This could possibly be a few days or maybe even a week! And would the worship period be this long?

One site gave the following information:

So, how long does each phase of the Moon last? Well, the phases are just names we give to certain points along the Moon's smooth path around Earth. Technically, each phase, just like the one called a New Moon, when the Moon is exactly between Earth and the Sun, lasts only a brief instant. But to our eyes, a New Moon can last for a few days, representing the time that the Moon appears in the sky too near the Sun's position for us to see it at all. The time it takes the Moon to go through all its phases is about a month, and that was so important to our ancestors that they created the period of time we call a month. Maybe you've even noticed that the word month is like the word moon.
http://spaceplace.nasa.gov/en/kids/phonedrmarc/2004_march.shtml
See Quote Note 1.

So what we call the new moon could last a few days. Is this the period of worship though?
Searching new-moon in the Old Testament brought to light two verses that resolved this problem. Though the quote by Isaiah possibly suggests a day these verses made it definite. The verses were Ezekiel 46:1 and 6:

Thus saith the Lord GOD; The gate of the inner court that looketh toward the east shall be shut the six working days; but on the sabbath it shall be opened, and in the day of the new moon it shall be opened.
And in the day of the new moon it shall be a young bullock without blemish, and six lambs, and a ram: they shall be without blemish.

We are obviously not interested in any sacrifices mentioned with the new-moon but nevertheless these verses give us the length of time: the day of the new moon. So the new moon worship period is one day in length, basically as expected but this was now conclusive.

The next step would be to get some sort of confirmation that I was on the right track. The obvious spot to check was Jewish sites that might mention this.

I very quickly ran into the term “Rosh Chodesh” which appeared to be the Jewish worship day for the new moon or beginning of the month. At this time I also found out that the Jewish month starts with the new moon. I was also going to find out that this was a very loose statement.

What is Rosh Chodesh?

Rosh Chodesh is the monthly celebration of the New Moon, according to the Jewish calendar. The Jewish calendar follows lunar months, each with 29 or 30 days, although the year is solar. Some scholars believe that lunar months derive from ancient nomadic calendars and solar years are the invention of agricultural societies; the Jewish calendar combines the two. Many Jewish festivals are tied to the lunar cycle; for example Sukkot and Passover begin on the full moon, in the middle of the month. Since 12 lunar months do not add up to one complete solar year, additional “leap months” are intercalated into the calendar in seven years out of a 19-year cycle.

Long ago, the appearance of the new moon each month was attested by witnesses. Once their testimony was deemed credible, fires were set on the hilltops to announce the new month to neighboring communities who, in turn, passed the message along. This system proved both dangerous and cumbersome, and once Jews lived outside Eretz Yisrael, it was wholely inadequate.

The present Jewish calendar was introduced in the time of Hillel II (358/9 CE), at which time astronomical calculations replaced the practice of calling witnesses before the Sanhedrin. Since that time, it has been possible to calculate the Jewish calendar well into the future on the basis of scientific calculations.
Source: Rabbi Scheinerman's homepage
http://www.jewishvirtuallibrary.org/jsource/Judaism/Rosh_Chodesh.html
See Quote Note 2.

This looked very promising and straightforward. The exact astronomical time of the new moon would give the day for worship and the first day of the Jewish month. But once I started looking at Jewish calendars on the internet I found this was anything but the truth. The first calendar I pulled up was:


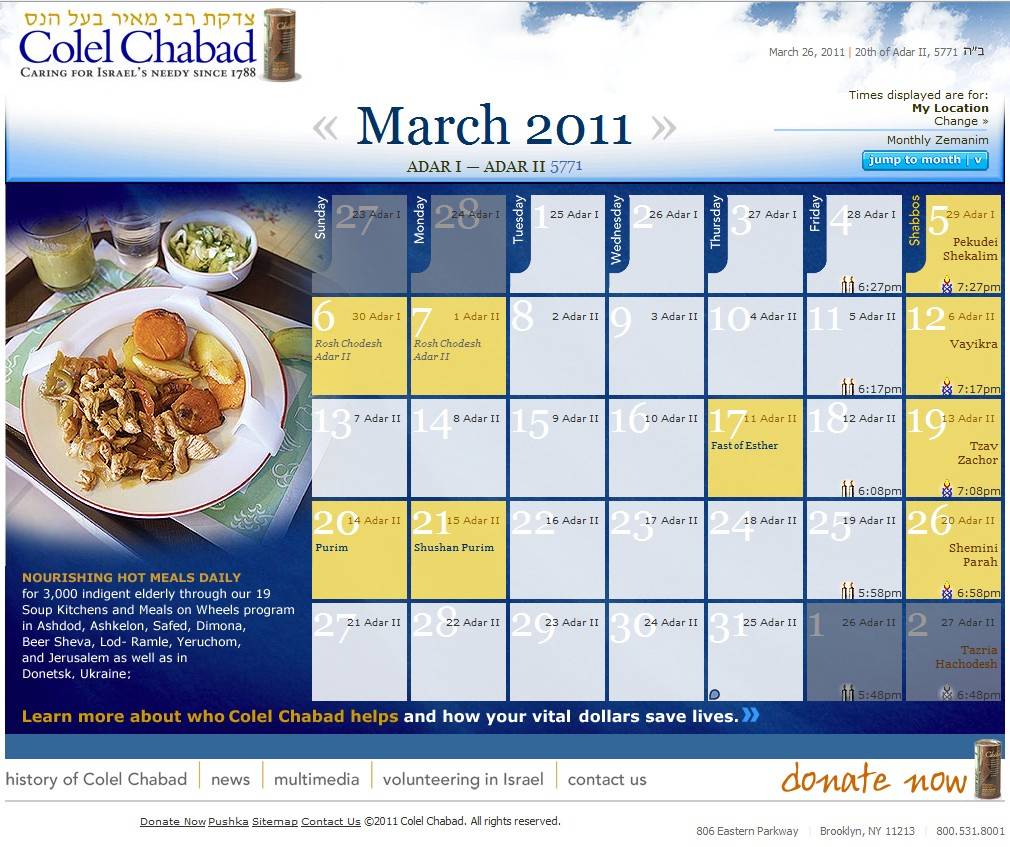
From https://www.colelchabad.org/Calendar.htm

The first thing I noticed there were TWO days listed as Rosh Chodesh for March 2011: Sunday 6 AND Monday 7!
I sent an e-mail message to this group about this and received a reply that answered the query about two Rosh Chodesh days. Part of their reply made this a bit clearer:

5. The Hebrew New Month, as it goes according to this calculation is either on the 30th or 31st day each month, as a month is 29.5 days, we have alternating New Months, being the 30th (and) or 31st day.
6. So one month the New Month is celebrated on the 30th day, and the month after it is (usually) celebrated on the 30th and 31st day.
Reply from colelchabad.

The following quotation [found at a later time and added here for continuity] also helps explain this:

Sometimes Rosh Chodesh is one day, but sometimes it is celebrated for two days. Months are based on the lunar cycle, of course. The amount of time required for the moon to make one complete revolution around the earth is determined by the conjunction of the earth, moon, and sun (i.e. they lie along a line). When this happens, it is the new moon. A complete revolution takes 29 days, 12 hours, 44 minutes, 3-1/2 seconds. Days in our calendar must begin at sundown, regardless of when the conjunction actually takes place. Hence it is necessary to either add or subtract a half a day from each calendar month. For this reason, Hebrew months alternate between 29 and 30 days in length, with the actually moment of the new moon falling in between.
Source: Rabbi Scheinerman's homepage
http://www.jewishvirtuallibrary.org/jsource/Judaism/Rosh_Chodesh.html
See Quote Note 2.

The second thing I noticed is that the day for the new moon did not sit well with my other chart obtained from the internet. For Sydney times I had the new moon in March on Saturday the 5^th at 7:46am. This would also be daylight saving time for where I was in Australia. The site I obtained the chart from also had UTC times. This gave the new moon on March 4 at 8:46pm. This was even worse and further away from the Jewish dates. I was not sure on the UTC times so decided to look further. And I couldn't have done better. I found a page from NASA which gave moon phases from 2001 to 2100. This of course was a long page and the part of interest with top of page is as follows:



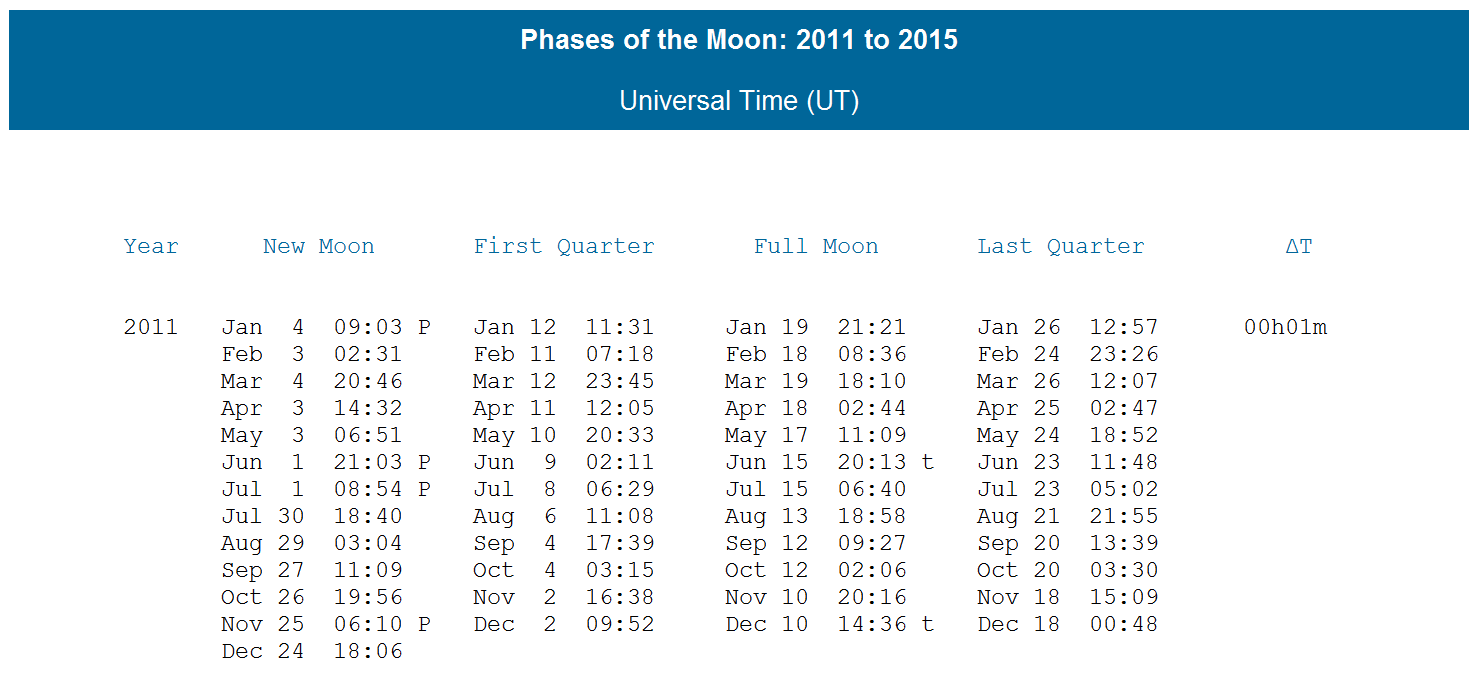
http://eclipse.gsfc.nasa.gov/phase/phases2001.html
See Quote Note 3.

This did help a lot. The NASA site also explained that “Universal Time is equivalent to Greenwich Mean Time or GMT.” So I could use the chart to determine any new moon times on the planet anywhere. Since the NASA chart gave the same times as my original UTC chart I could then use either one. For Sydney Australia it was simply a matter of adding 10 hours plus one for daylight saving. But it didn't help the Jewish calendar Rosh Chodesh dates I was finding. I wrote this time to a couple of different Jewish groups in Sydney about this:

I have had a reply from another Jewish group about the New Moon but there is still something wrong with the dates.
By NASA the last New Moon was on Saturday 7:46 AM Sydney Australia time.
The NASA universal time is given as Mar 4 20:46. To get to us [Sydney, Australia time] we add 10 hours plus one hour for daylight saving giving
20:46 + 10 + 1 = 31:46 and correct this to 7:46 the following day or Saturday morning Mar 5.
This day and time agrees with other web sites giving Moon information for Sydney Australia.
Anyway your site gives the New Moon day as Mon 7 Mar.
Another Jewish site gives Mar 6 AND Mar 7.
They explained this by saying the count of days for the Jewish month is 29.5 days so every few months they count the second last day AND the last day of the month.
But nomatter which way, for the last New Moon the Jewish choice of Mar 6 and/or Mar 7 still is not correct if the actual New Moon was on Sat Mar 5 [Sydney, Australia time].

I have had no reply.

Anyway about this time I was also looking on the internet to check the lunar month time and had also worked out a rough approximation using the first date chart I obtained. For February to October I worked out 10 periods and took the average and got something round 29.53 days ignoring the minutes. I think with the minutes this went to about 29.54. These figures are reasonable and are in the range given by NASA top of page previous.

A little more information about this... In one of the replies was a comment:

We have a New Moon every 29 days, 12 hours and 44 1/18 minutes, so once you have the new moon for any given month you can figure every future one by adding the 29.12.44.1/8.

Now this is not exactly correct. Apparently the period changes through the yearly cycle but over a reasonable period of time the average would give the value quoted. I decided to plot and average the values for 2011 with results as follows.
Note: the lunar month with approximate period 29.53 days is called the synodic month. This explains the titles in the following table and plot. These were done at a later time in this study and added here for continuity.

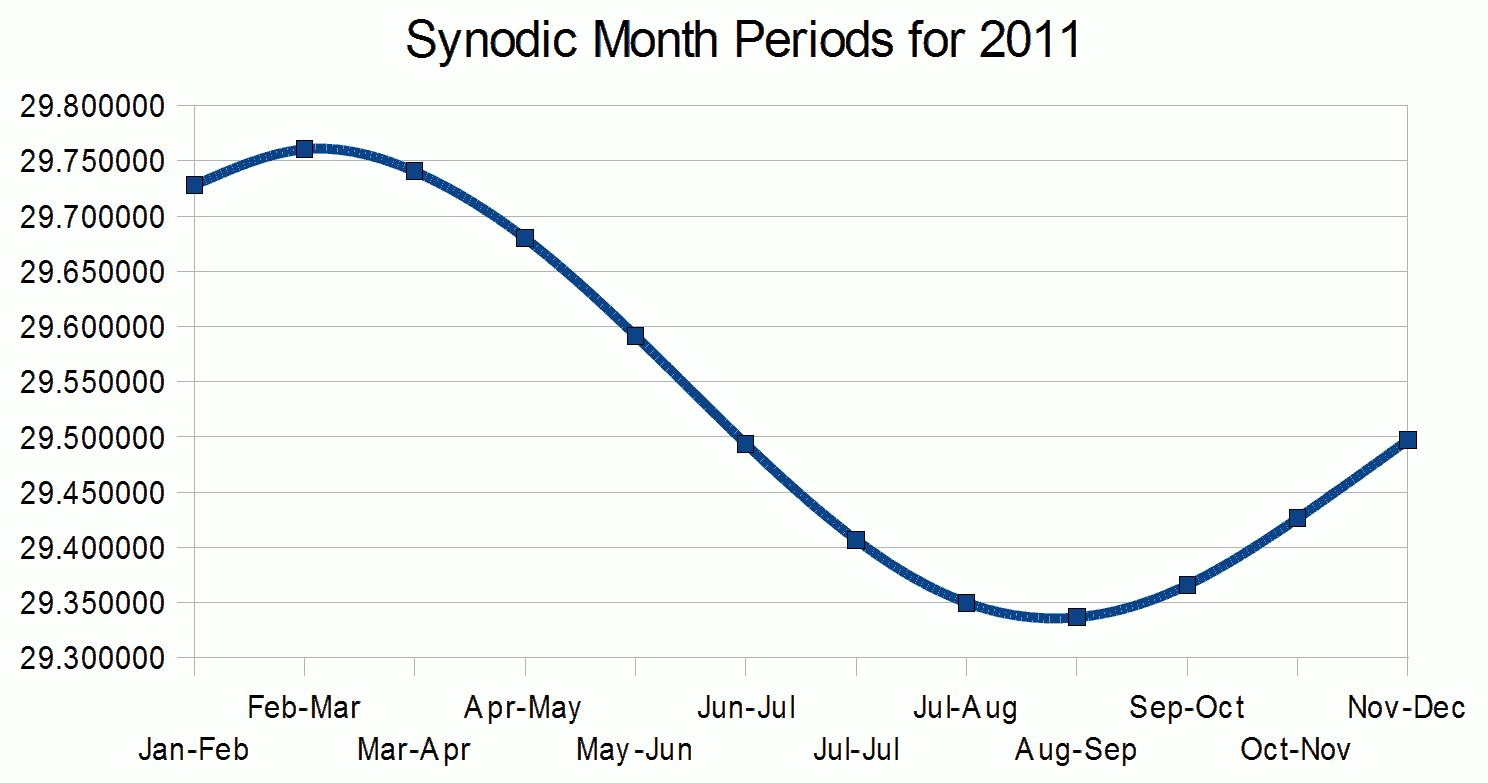
Through 2011 the synodic month length ranged from 29.34 days up to about 29.76 days.
The maximum value minus the minimum value is about 0.42 days or approximately 10.1 hours.
So we could say that the period for the synodic month any month of 2011 could be considered to be approximately 29.53 days plus or minus 5 hours. It could be plotted. Interestingly if you could determine approximately where you were on the graph, you could have a good guess at the next moon phase date you were investigating. For anyone doing visual sightings this graph could be extremely important.
Note: the synodic month being shorter than most calendar months will sometimes fall inside a month: there were two new moons in July, hence Jul-Jul on the axis.

The other thing that happened here I became aware of two different month periods. There are actually a few but probably the two most well known ones are the sidereal and synodic months.

A synodic month is 29.53059 days (29 days, 12 hours, 44 minutes, 2.8 seconds) and is measured from New Moon to New Moon.
http://en.wikipedia.org/wiki/Lunar_month
See Quote Note 4.

So the synodic month is actually what we are interested in here and should be the same in theory as the Jewish month.

The Moon's orbital period in a non-rotating frame of reference (which on average is equal to its rotation period) is about 27.32166 days (27 days, 7 hours, 43 minutes, 11.6 seconds). This is known as a sidereal month and is measured by observing how long it takes the Moon to pass a fixed star on the celestial sphere.
http://en.wikipedia.org/wiki/Lunar_month
See Quote Note 5.

Simply put, the sidereal month is the time it takes the Moon to complete one orbit around the Earth.

Wait a minute! Isn't one orbit of the moon around the earth meant to bring the moon from one new moon to the next new moon? So shouldn't these two periods be the same in length? Why are they different?

A synodic month is longer than a sidereal month because the Earth-Moon system is orbiting the Sun in the same direction as the Moon is orbiting the Earth. Therefore, the Sun appears to move with respect to the stars, and it takes about 2.2 days longer for the Moon to return to the apparent position of the Sun. The synodic month is the most common way of expressing the lunar cycle.
http://en.wikipedia.org/wiki/Lunar_month
See Quote Note 6.

To my shame I have to admit I have not really run across this before or even given it much thought—until now, after having read about it in the investigation of this matter. The best news is that it is actually very easy to show using a diagram. The mathematics of it is really very simple. Thankfully. This sort of thing as you could imagine could get very messy very quickly. In this case it doesn't!

It can be done very simply by approximating the orbit of the earth as circular. Now someone is going to say you can't do that—the Earth's orbit is elliptic. True, but the eccentricity of the orbit makes it nearly circular:

The eccentricity of the Earth's orbit is currently about 0.0167, meaning that the Earth's orbit is nearly circular, the semiminor axis is 98.6% of the semimajor axis.
http://en.wikipedia.org/wiki/Orbital_eccentricity
See Quote Note 7.

The eccentricity of an ellipse, usually denoted by ε or e, is the ratio of the distance between the two foci, to the length of the major axis or e = 2f/2a = f/a. For an ellipse the eccentricity is between 0 and 1 (0 < e < 1). When the eccentricity is 0 the foci coincide with the center point and the figure is a circle.
http://en.wikipedia.org/wiki/Ellipse
See Quote Note 8.

Actually this approximation works pretty well and it can be used to evaluate the Moon's orbital sidereal month given the length of the synodic month!

Here's a diagram I have constructed to show this [nothing to scale of course]:

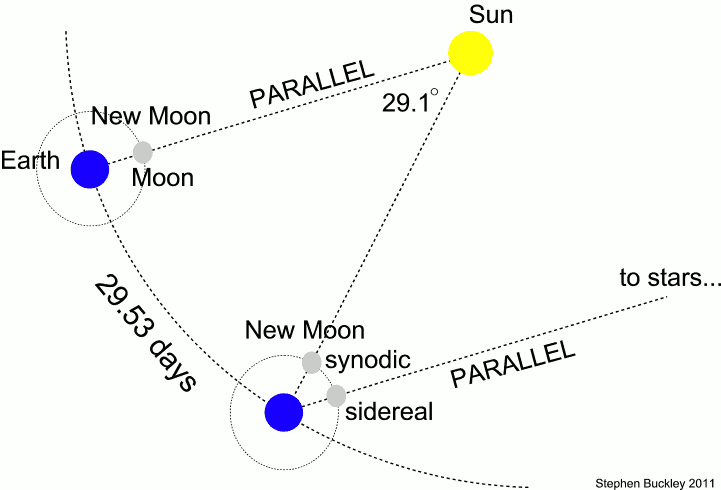
The diagram shows the moon positions for one synodic and sidereal month. The Earth position is for one synodic month but for a simple comparison the moon position for the sidereal month is shown at the same time. As you can see from the diagram the moon has to travel some extra distance beyond a single orbit of the Earth to get to the next new moon. We can work out the extra angle and time as follows.
Note: the sidereal moon position shown above would be of course at approx 27.32 days.

To work out the angle of travel of the Earth we use the length of the synodic month of approximately 29.53 days. The angle of travel is simply what this gives given the year of 365.25 days marking out 360 degrees for the orbital circle [approximating the ellipse]. This calculation is simply:

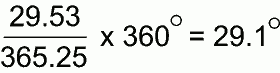
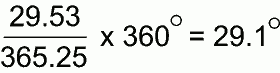
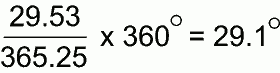
So as the moon travels from one new moon to the next the Earth travels approximately 29.1 degrees in its orbit around the Sun.
For the parallel lines this gives the same angle the moon must travel to get to the next new moon after one complete orbit of the Earth. The moon also has to travel an extra 29.1 degrees!!

Further, it is possible to use this result to find an approximate calculation for the sidereal month given the synodic month is 29.53 days.
The approximation is as follows. Since for the synodic month the moon must travel through 360 + 29.1 degrees, then to travel only one orbit requires just 360 degrees giving:



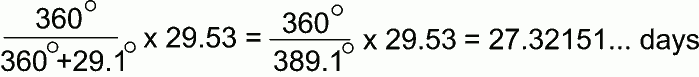
This approximate calculation agrees with the true sidereal month value to 5 significant digits. That's a pretty good approximation!
Similarly the approximation can be used to calculate the difference in the sidereal and synodic months as follows. Since the moon must travel 289.1 degrees from new moon to new moon then the difference can be determined as:



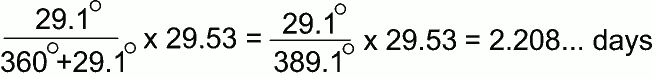
or approximately 2.2 days as stated in the previous quotation.

Before continuing with the new moon investigation there is a very important question to ask here. What is the obvious question to ask from looking at the diagram? Have a quick look at it again. Note especially the new moon positionings. Just what else do you think of when the moon is directly between the Earth and the Sun?

Answer: when the moon is directly between the Earth and the Sun would not that give us a solar eclipse? If we have a new moon every 29.53 days why don't we have a solar eclipse then too? It's a good question and struck me when I was reviewing this study. I looked for the answer and found the following information:

The Moon is a cold, rocky body about 2,160 miles (3,476 km) in diameter. It has no light of its own but shines by sunlight reflected from its surface. The Moon orbits Earth about once every 29 and a half days. As it circles our planet, the changing position of the Moon with respect to the Sun causes our natural satellite to cycle through a series of phases:

• New Moon > New Crescent > First Quarter > Waxing Gibbous > Full Moon > Waning Gibbous > Last Quarter > Old Crescent > New Moon (again)

The phase known as New Moon can not actually be seen because the illuminated side of the Moon is then pointed away from Earth. The rest of the phases are familiar to all of us as the Moon cycles through them month after month. Did you realize that the word month is derived from the Moon's 29.5 day period?

To many early civilizations, the Moon's monthly cycle was an important tool for measuring the passage of time. In fact many calendars are synchronized to the phases of the Moon. The Hebrew, Muslim and Chinese calendars are all lunar calendars. The New Moon phase is uniquely recognized as the beginning of each calendar month just as it is the beginning on the Moon's monthly cycle. When the Moon is New, it rises and sets with the Sun because it lies very close to the Sun in the sky. Although we cannot see the Moon during New Moon phase, it has a very special significance with regard to eclipses.

An eclipse of the Sun (or solar eclipse) can only occur at New Moon when the Moon passes between Earth and Sun. If the Moon's shadow happens to fall upon Earth's surface at that time, we see some portion of the Sun's disk covered or 'eclipsed' by the Moon. Since New Moon occurs every 29 1/2 days, you might think that we should have a solar eclipse about once a month. Unfortunately, this doesn't happen because the Moon's orbit around Earth is tilted 5 degrees to Earth's orbit around the Sun. As a result, the Moon's shadow usually misses Earth as it passes above or below our planet at New Moon. At least twice a year, the geometry lines up just right so that some part of the Moon's shadow falls on Earth's surface and an eclipse of the Sun is seen from that region.
http://www.mreclipse.com/Special/SEprimer.html

Admittedly we only needed the last paragraph but there was a wealth of information here that just could not be missed; will mention some of this again later. Curiously though this site has the orbit of the moon as the synodic length, not the sidereal. A simple mistake.

Now back to the investigation...

I was still investigating the Jewish sites on the internet trying to make sense of all this why their dates for Rosh Chodesh did not seem to fit with the astronomical information I was finding.
The Hebrew word in the Bible translated as the new moon is Chodesh and Brown, Driver, and Briggs give information on this word:

H2320
חדשׁ
chôdesh
Brown, Driver, and Briggs' Definition:
1) the new moon, month, monthly
1a) the first day of the month
1b) the lunar month

2320 is the Strong's Concordance number for this word. The H before the number above just signifies this is a Hebrew word since there are also Strong's numbers for Greek words from the New Testament. You can see the Hebrew word for this below the number above and it is read from right to left and would be pronounced, of course, as “chodesh.”

So why do all the Jewish sites refer to Rosh Chodesh instead of just Chodesh? I kept searching and found the answer.

Opening Ceremony

We are gathered to celebrate Rosh Chodesh, the beginning of the new Jewish month. When introducing the commandment of Rosh Chodesh, the Torah says: "This month shall mark for you the beginning of the months; it shall be the first of the months for you" (Exodus 12:2). Commenting on the fact that the verse says "This month shall mark for you," Rabbi Samson Raphael Hirsch writes: "This renewal of the moon shall be a beginning of renewals for you. Noticing, realizing the fresh birth of the moon shall induce you to achieve a similar rejuvenation. You are to fix your moons, your periods of time by taking note of this ever fresh recurring rejuvenation." Rosh Chodesh is a time for introspection and reflection on the previous month that was, and on the new month that will be.
http://www.hillel.org/jewish/rituals/roshchodesh/open_close.htm
See Quote Note 9.

This does appear to be where the term came from. Looking at Exodus 12:2:

This^H2088 month^H2320 shall be unto you the beginning^H7218 of months:^H2320 it^H1931 shall be the first^H7223 month^H2320 of the year^H8141 to you.

You can see the Strong's number H2320 translated here as months. That's Chodesh. The Hebrew word before it, H7218 is:

H7218
ראשׁ
rô'sh
Brown, Driver, and Briggs' Definition:
1) head, top, summit, upper part, chief, total, sum, height, front, beginning
1a) head (of man, animals)
1b) top, tip (of mountain)
1c) height (of stars)
1d) chief, head (of man, city, nation, place, family, priest)
1e) head, front, beginning
1f) chief, choicest, best
1g) head, division, company, band
1h) sum

So together these two words give Rosh Chodesh and mean the beginning of the month.
Now from here things get a little hazy.

So far my investigation was aimed at looking at the day of the new moon, and when was it. To my satisfaction I had been completely successful in this attempt. But now things were about to get a whole lot worse before they got better. Get better they did and I did eventually completely resolve this muddle, to my satisfaction, at least.

But what went wrong?

You have a good idea so far. None of the astronomical new moon dates I was finding seemed to fit any of the Jewish calendars. Then I started to find pictures like the following on some of the Jewish sites:


See Quote Note 10

Look at the picture. Note the title on the right hand side says: ROSH CHODESH: A New Moon.

Well maybe this is what the new moon looks like to the Jewish mind but to me I thought it looked more like the phase following the new moon. So the waters may be muddying a bit here. Digging a bit deeper I found the following:

According to the Mishnah and Tosefta, in the Maccabean, Herodian, and Mishnaic periods, new months were determined by the sighting of a new crescent, with two eye witnesses required to testify to the Sanhedrin to having seen the new lunar crescent at sunset. The practice in the time of Gamaliel II (c. 100 CE) was for witnesses to select the appearance of the moon from a collection of drawings that depicted the crescent in a variety of orientations, only a few of which could be valid in any given month.
http://en.wikipedia.org/wiki/Hebrew_calendar#Importance_of_lunar_months
See Quote Note 11

So here we are told the new month was determined by the sighting of the new lunar crescent at sunset. The next quote gives some information about the judicial process for these sightings:

An essential element of the Jewish calendar is the determination of Rosh Chodesh, the day when each month begins. Since our months, by biblical law, begin with the sighting of the new moon (molad), which can occur on either the thirtieth or thirty-first night of a given month, we need to determine which of these is Rosh Chodesh. In ancient times, this was done through eyewitnesses, who would testify before the High Court in Jerusalem that they had seen the new moon the previous evening; once they had been cross-examined and judged to be trustworthy, the court would declare the day to be sanctified (Mekudash) as Rosh Chodesh. This process is known as Kiddush Ha-chodesh (sanctification of the month). Nowadays, we have a fixed calendar, and we observe either one day of Rosh Chodesh (if the thirtieth day becomes the first of the new month) or two (the thirtieth and the thirty-first, the latter becoming the first day of the new month). Nevertheless, on the Shabbat preceding Rosh Chodesh (for all months but Tishrei), we make a formal announcement in the synagogue about it, amid prayers for peace and prosperity in the coming month, which we call Birkat Ha-chodesh (blessing of the month). What is the relationship between them? The Magen Avraham writes (OC 417:1):

The customary practice is to stand while proclaiming that Rosh Chodesh will be on such-and-such a day, similar to Kiddush Ha-chodesh, which was performed standing.

Rav Akiva Eger raises an objection (Hagahot, ad loc.):

I am ignorant and do not know where we find that Kiddush Ha-chodesh was performed standing. On the contrary, [what is stated] at the beginning of Rosh Ha-shana implies that it was performed seated.

Rav Akiva Eger appears to be referring to the first mishna in the third chapter of Tractate Rosh Ha-shana:

If three people saw [the molad], and they are the court – two should stand up, seat [two] of their colleagues next to the third, and testify before them, and they say: "Mekudash! Mekudash!"

Even without this proof from Rosh Ha-shana, it seems to be clear that the court must be seated when it sanctifies the month, for all judicial actions require that the judges be seated, and Kiddush Ha-chodesh is a judicial action!
http://vbm-torah.org/archive/halak68/19halak.htm
See Quote Note 12.

For any non-Jews reading this the last quote may even seem quite humorous. Nevertheless, the importance of this last quote cannot be underestimated. It introduces something completely new. The molad.

What is the molad?

The Jewish calendar is lunar-based, with each month representing one lunar cycle -- the time it takes for the moon to complete one orbit around the earth.
The molad is the time of the moon's "birth." There is a point in the moon's orbit in which it is positioned directly between the earth and the sun, making it invisible to anyone standing on earth's surface. The molad occurs when the moon has moved far enough from this position that a thin crescent of its illuminated surface becomes visible, marking the start of a new Jewish month.
The time it takes for the moon to complete one orbit around the earth -- as calculated by sages and confirmed by astronomical observation -- is 29 days, 12 hours and 793 chalakim (there are 1,080 chalakim or "parts" in an hour, hence a chelek is 3.33... seconds). This represents the average time from molad to molad. The actual moment at which the moon becomes visible will vary slightly from the average molad, depending on the relative positions of the earth, moon and sun to each other at any given month, where on earth the observer is standing, the length of twilight at given seasons of the year, and other astronomical variables.
For this reason the Jewish calendar is “tweaked” so that Rosh Chodesh -- the first of the month -- is as close as possible to the time in which the moon would actually be seen in Jerusalem if we were still relying on actual observation of the moon to establish the start of a new month (as was done until the 5th century C.E.). Also, because a month has to be made up of whole days, the Jewish month alternates between 29 and 30 days. This is why Rosh Chodesh will often occur a day or more after the average molad.
http://www.chabad.org/library/article_cdo/aid/216238/jewish/Molad-Times.htm#notes

Well, this is interesting. The lunar month for the moon to complete one orbit around the Earth is the sidereal month of approximately 27.32 days, not the synodic month which is wanted here. The synodic month would give the correct time from first thin crescent to the next first thin crescent. And we know this is the same as from new moon to new moon. It's an easy mistake to make and we would not condemn the writer of the above article for making it.

This last quotation is telling us that Chodesh is determined as the day the thin crescent appears after the new moon. Another important point that we now have is that Rosh Chodesh should be determined for the Jerusalem time zone. I may disagree with this later but for continuity and for trying to align my results so far with the Jewish calendar we will take this approach here.

Later found another site that confirms above more clearly; that is, the crescent approach, and Jerusalem as the base position for determining Chodesh for the Jewish calendar:


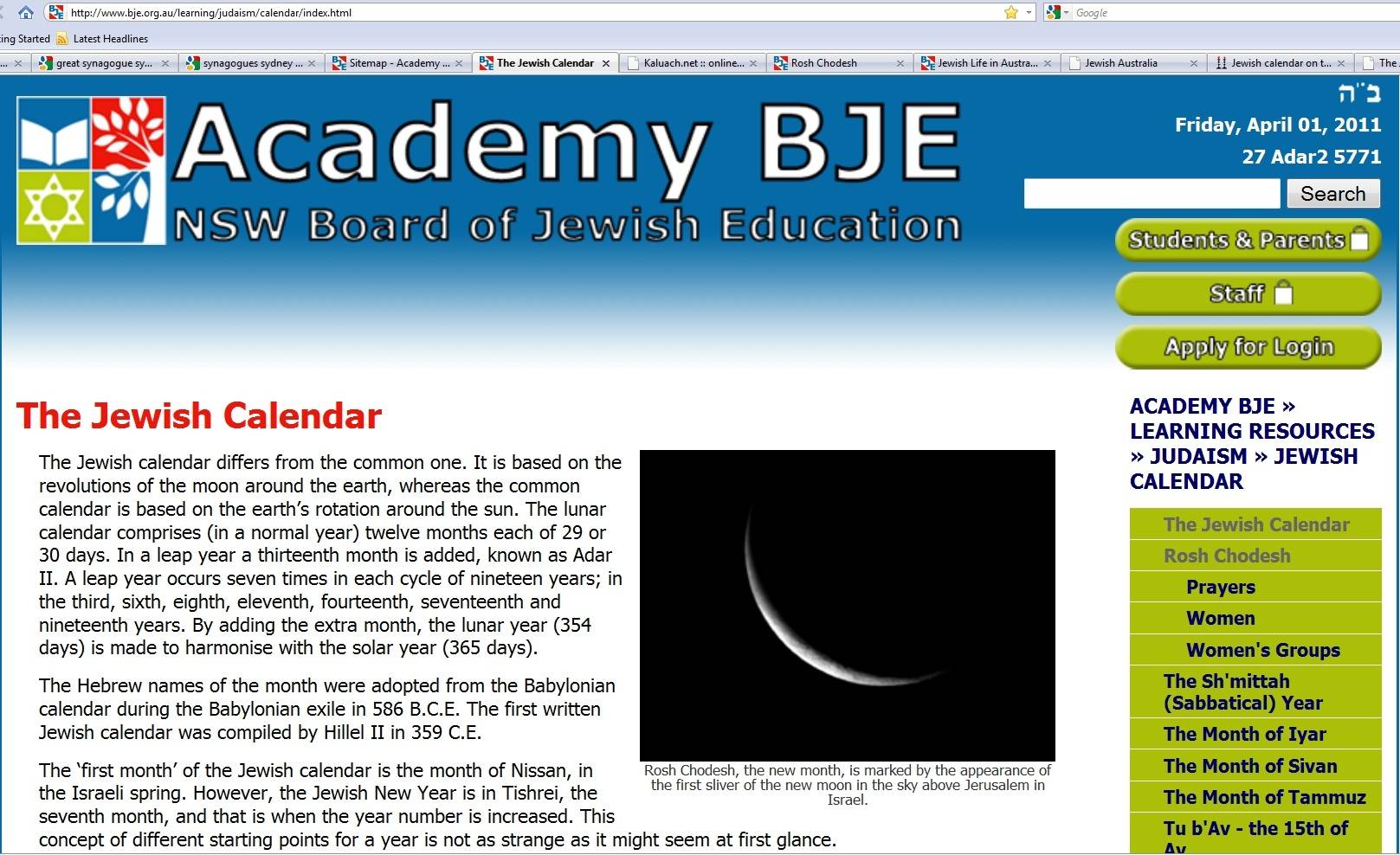
http://www.bje.org.au/learning/judaism/calendar/index.html
See Quote Note 13

Note the text under the picture: “Rosh Chodesh, the new month, is marked by the appearance of the first sliver of the new moon in the sky above Jerusalem in Israel.”

This says it all in one sentence!

The time correction for Jerusalem can be found from any map showing time zones. I found one as follows:


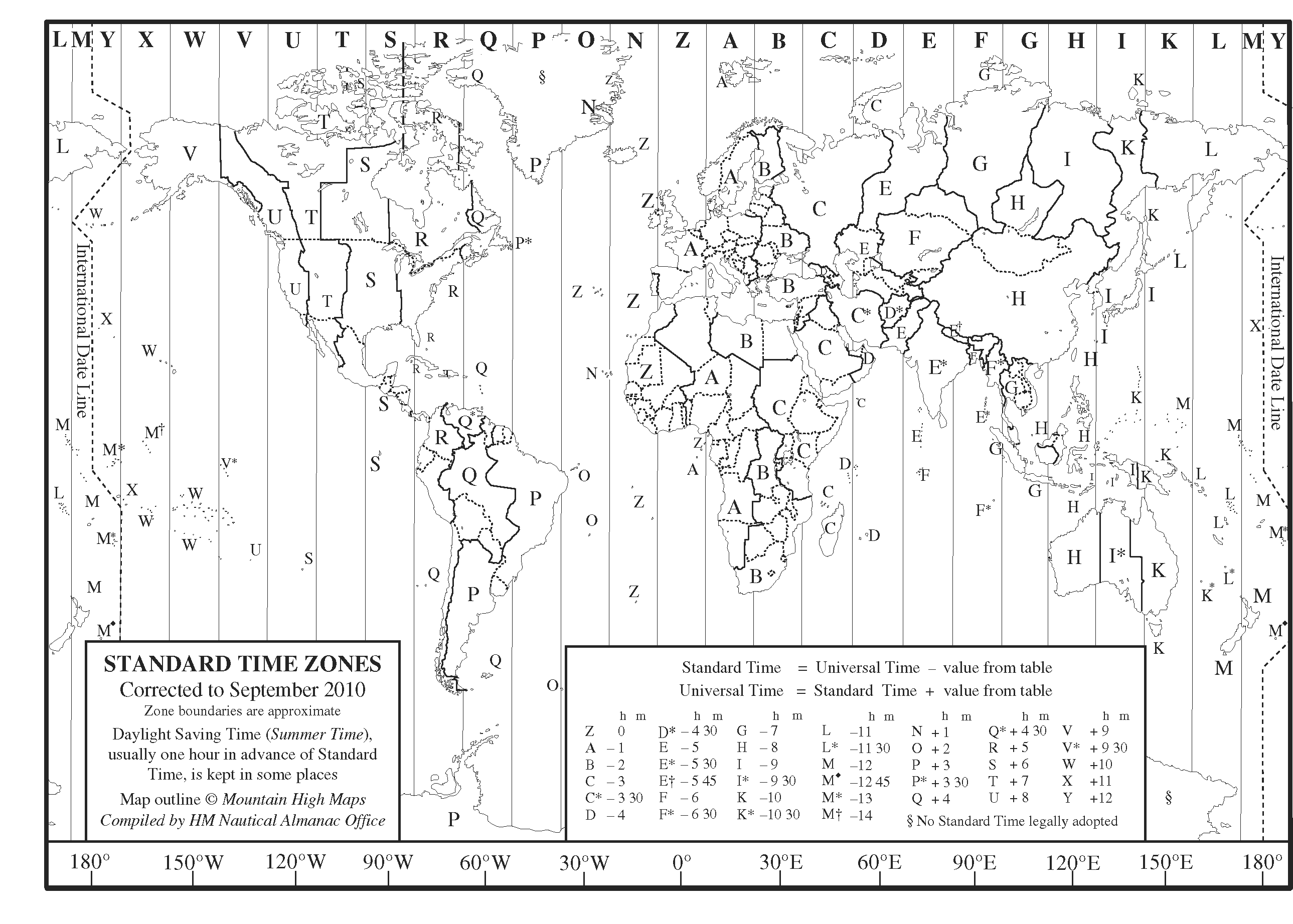
From http://aa.usno.navy.mil/faq/docs/world_tzones.php
See Quote Note 14

Jerusalem would be in zone B. Sydney Australia is in zone K and the above chart gives Universal Time − (−10) = GMT + 10 which looks correct. So the correction for Jerusalem would then be GMT + 2. This looks right.
The NASA chart gave the universal time for the New Moon in March as Friday March 4 20:46. Adding 2 hours takes this to March 4 22:46 or 10:46pm still on Friday. The Jewish calendar worship days would most likely be adjusted from sunset to sunset [we mention this later in this investigation]. So if we take this time as the correct time to determine Rosh Chodesh the Jewish calendar would probably have Rosh Chodesh as Saturday March 5 as the 10:46pm time is after sunset.
Well this still hasn't got us to March 6 or 7 as the other Jewish calendars I looked at showed. The above calculation was done using the astronomical new moon. The crescent approach would be a day or two later and would fit the Jewish calendars.

Some more on the crescent:

In parashat hashavua (weekly Torah reading) Bo, we read: “Then Adoshem said to Moses and to Aaron in the land of Egypt, saying: this renewal of the moon shall be for you the beginning of new moons; it shall be to you the first of the months of the year.” (Exodus 12:1-2) And we note that Akeidat Yitzchak (Rabbi Yitzchak ben Moshe Arama, 1420-1494) teaches that this was the first commandment we received as a nation, while we were still in Egypt.
Here’s the picture: Adoshem calls Moses and Aaron and shows them the silver crescent of the moon. ... The sign signifies a beginning of renewals or revivals because, according to Rabbi Samson Rafael Hirsch (1808-1888), chodesh doesn’t mean month but the beginning of the month. It is a day that we are to consecrate, using it to separate and dedicate ourselves to a sacred purpose. The new moon is somehow to be an inducement to moral spiritual action in our day-to-day lives.
So while we have this commandment to sanctify the beginning of the months, it isn’t about commemorating astronomical events, such as the phases of the moon. This isn’t a form of nature-worship.
ROSH CHODESH A VITAL HOLY DAY
By Rabbi Moshe ben Asher, Ph.D. & Magidah Khulda bat Sarah
See Quote Note 15.

By the time I got to the end of all this I was really puzzled. Who was right? Did the month start with the new moon or this first thin crescent? Could I go by the Bible references to the new moon? After all, the prophet Isaiah conclusively states from one new moon to another. That should be the end of the matter. Or is it? The problem may be the translation. The Hebrew word translated new moon is Chodesh. But these Jewish quotations are all saying they go looking for chodesh by searching for this first thin crescent. In other words, it's their spin on it and how they have translated the instructions the Lord gave Moses in Exodus chapter 12.

I was struggling here with how the Jews were interpreting the instructions given to them about thirty five hundred years ago. Simply put, our current understanding of these matters, new moon terminology etc just may not be sufficient to sort this out. The only way to resolve this problem was to go back to the early part of the Bible and see if it was possible to determine just how the month was supposed to be constructed. Taking this approach I was actually able to find an answer. We proceed as follows...

The moon was given for seasons:

And God said, Let there be lights in the firmament of the heaven to divide the day from the night; and let them be for signs, and for seasons, and for days, and years:
And let them be for lights in the firmament of the heaven to give light upon the earth: and it was so.
And God made two great lights; the greater light to rule the day, and the lesser light to rule the night: he made the stars also.
And God set them in the firmament of the heaven to give light upon the earth,
Genesis 1:14-17

The word translated as season is:

H4150
מוֹעָדָה מֹעֵד מוֹעֵד
mô‛êd mô‛êd mô‛âdâh
mo-ade', mo-ade', mo-aw-daw'
From H3259; properly an appointment, that is, a fixed time or season; specifically a festival; conventionally a year; by implication, an assembly (as convened for a definite purpose); technically the congregation; by extension, the place of meeting; also a signal (as appointed beforehand): - appointed (sign, time), (place of, solemn) assembly, congregation, (set, solemn) feast, (appointed, due) season, solemn (-ity), synagogue, (set) time (appointed).

We would read this as a fixed time, a period of time. Note it states conventionally a year. But years is already mentioned in the verse so this period of time is not a year. But the word seems to be more saying a set time instead of a period. That is, the moon is marking a set time. Where else is this thought conveyed?

Psalm 104:19 gives:

He appointed the moon for seasons: the sun knoweth his going down.
KJV

and the Good News Bible gives this as:

You created the moon to mark the months; the sun knows the time to set.

Now lets have a look again at the main verse the Jewish sites refer to for the beginning of months.
Exodus 12:2 gives:

This month shall be unto you the beginning of months: it shall be the first month of the year to you.

The three words in this verse translated month are all chodesh. This is clearly not where months began for they are mentioned well before this. The statement in Exodus chapter 12 is just laying out where the Jewish year started. This month shall be the first month of the year. But it could also be translated as new moons for the year. This is the new moon, giving the implication that the new moon is also the beginning of each month.

Another interesting point about month:

A month is a unit of time, used with calendars, which was first used and invented in Mesopotamia, as a natural period related to the motion of the Moon; month and Moon are cognates. The traditional concept arose with the cycle of moon phases; such months (lunations) are synodic months and last approximately 29.53 days.
http://en.wikipedia.org/wiki/Month
See Quote Note 16.

So here is another point usually missed. Moon and month are cognates:

In linguistics, cognates are words that have a common etymological origin. The word derives from the Latin cognatus (blood relative).
http://en.wikipedia.org/wiki/Cognate
See Quote Note 17.

So the term new moon could also mean new month. That's pretty clear.

From the verse it does sound like the new moon is the beginning of the month. Is there any other way to determine this?
Consider the construction of the day. In Genesis chapter one the day is continually referred to:

Verse 5: And the evening and the morning were the first day.
Verse 8: And the evening and the morning were the second day.
Verse 13: And the evening and the morning were the third day.
Verse 19: And the evening and the morning were the fourth day.
Verse 23: And the evening and the morning were the fifth day.
Verse 31: And the evening and the morning were the sixth day.

As each day came from the hand of the Creator He stated that the day is classified as the evening followed by the morning. Of course by morning we don't literally mean just the morning say till about 12 mid day. The following two verses make this point very clear:

It shall be unto you a sabbath of rest, and ye shall afflict your souls: in the ninth day of the month at even, from even unto even, shall ye celebrate your sabbath.
Lev 23:32

And at even, when the sun did set, ...
Mark 1:32

The sabbath was the seventh day of the week and we could similarly apply the previous day length verses to give:

And the evening and the morning were the seventh day.

Of course the Bible doesn't say it in these words. The comment has been made for the previous six days of the week. And when we come to the second chapter of Genesis, the seventh-day is clearly mentioned.

Verse 2: And on the seventh day God ended his work which he had made; and he rested on the seventh day from all his work which he had made.

From my computer dictionary the meaning for sabbath is given as:

[Sabbath] A day of rest and worship: Sunday for most Christians; Saturday for the Jews and a few Christians; Friday for Muslims.
TheSage's English Dictionary and Thesaurus.

Anyway you look at this the sabbath is a day. And it is the seventh day of the week mentioned in Genesis chapter two. The day construct from Genesis chapter one gives the evening followed by the morning. The two hebrew words translated evening and morning are ereb and boquer. The Brown, Driver, and Briggs definitions for these days are:

H6153
ערב
'ereb
Brown, Driver, and Briggs' Definition:
1) evening, night, sunset
1a) evening, sunset
1b) night

and

H1242
בּקר
bôqer
Brown, Driver, and Briggs' Definition:
1) morning, break of day
1a) morning
1a1) of end of night
1a2) of coming of daylight
1a3) of coming of sunrise
1a4) of beginning of day
1a5) of bright joy after night of distress (figuratively)
1b) morrow, next day, next morning

So what the verses are saying is that the sunset comes first giving the start of the day. This is reflected in the verses previously quoted; Lev 23:32 and Mark 1:32:

from even unto even, shall ye celebrate your sabbath.
And at even, when the sun did set, …

So the full day period is defined as being from sunset to sunset. The following diagram shows this:



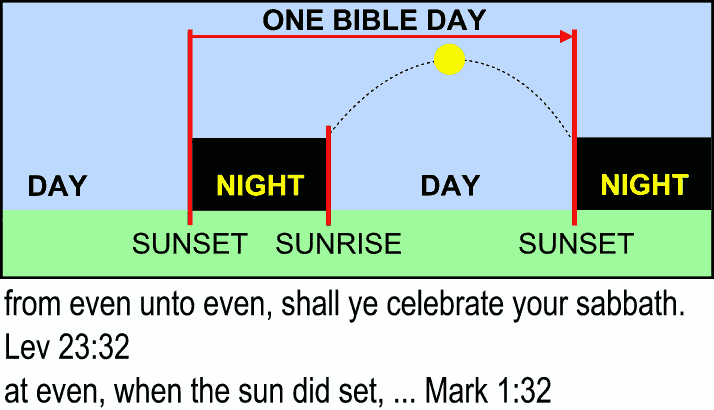
The important part to glean from this is that the dark part of the day comes first followed by the light part:



This is how the Creator has defined the day in Genesis.
We should be able to apply this approach to the moon and month. This would give the Creator's definition for the month. Have a look at the following phases of the moon as it moves through the month and ask yourself; from the previous understanding of how God constructed the day, just where would you start and end the month according to these phases?
Note: the moon pics here are shown for the southern hemisphere.



Well, it's pretty hard to get this wrong. The dark part must come first. According to sunset for the beginning of the dark part of the day the answer must simply be the start of the new moon. This would simply be determined as the day of the new moon. So the Bible month would be as follows:



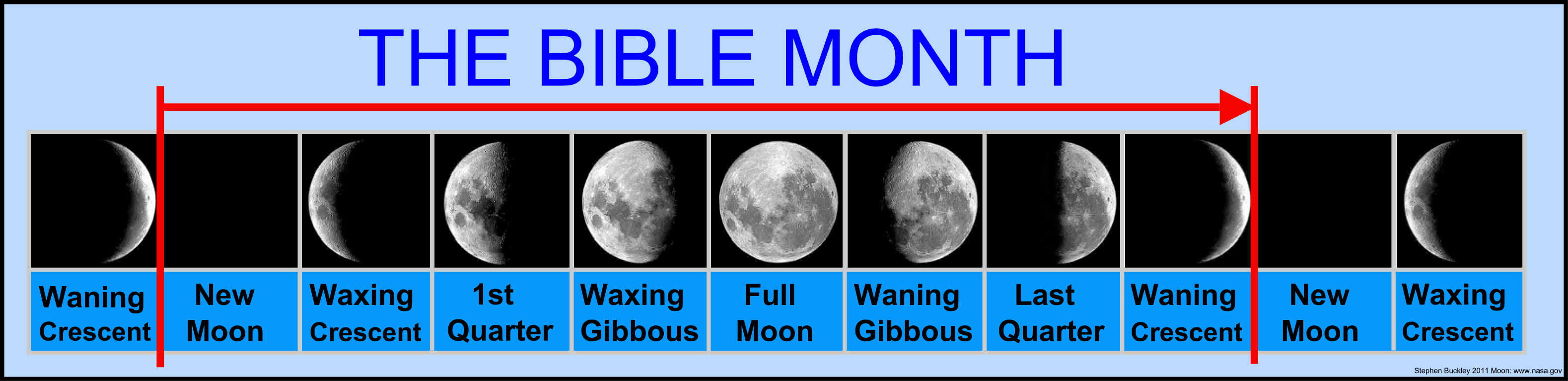
And it would appear to be the mind of God on this matter. Just about everywhere you look the month is defined as being from new moon to new moon; all the way from NASA down to the most general sites on astronomy:

the mean synodic month (New Moon to New Moon)
http://eclipse.gsfc.nasa.gov/phase/phases2001.html
See Quote Note 18.

The New Moon phase is uniquely recognized as the beginning of each calendar month
http://www.mreclipse.com/Special/SEprimer.html

A synodic month is 29.53059 days (29 days, 12 hours, 44 minutes, 2.8 seconds) and is measured from New Moon to New Moon.
http://en.wikipedia.org/wiki/Lunar_month
See Quote Note 4.

Lunar Month: The period between successive new moons (29.531 days).
TheSage's English Dictionary and Thesaurus.

lunar month. the period of a complete revolution of the moon around the earth, as the period between successive new moons (synodic month), equal to 29.531 days
http://dictionary.reference.com/browse/synodic+month
See Quote Note 19.

The month was originally defined as the time between one new moon to the next, a period now called the synodic month.
http://scienceworld.wolfram.com/astronomy/Month.html

Sometimes other phases of the moon are mentioned but the most common is the new moon.

Before continuing I have just been made aware of something very important that sheds more light on this and actually backs this premise up and reinforces it. It strengthens the argument here for the Lord's construction of the day. We have stated correctly that the construction for the day is sunset to sunset. That is how it is stated in the Bible. It is how the Lord defines a day. From sunset to sunset. I have applied this approach to the moon for the construction of the month. This is far more apt than originally thought. Here's the quote:

Many students get confused about the geometry of the orbit of the Moon because they have heard this term "the dark side" of the Moon (besides being a famous---at least to us old timers---album by Pink Floyd). If you examine the figures above about how the phases of the Moon change, you see that for at least one half of the lunar orbit, each spot on the Moon has daylight. That is there are 14.75 days when the Sun is visible each orbit, and 14.75 days each orbit when the Sun is not visible. At any one time (just like on the Earth!!!), there is one half of the Moon which is in darkness, and one half which is in the sunlight. It is true that the Moon spins much more slowly than the Earth, but the Moon has "days" and "nights". It is just that these days and nights are 14.75 Earth days long! So the far-side of the Moon which we cannot see is not the "dark side", it is the far-side. At full Moon, the far-side is completely dark. But at New Moon, the far-side is fully illuminated (as seen from the Sun!).
http://ganymede.nmsu.edu/tharriso/ast110/class05.html

What is the point of this quote? We only see one side of the Moon. Why? Because the Moon is also rotating on its axis. The time the Moon has to do “one orbit” about its axis takes the same time as one synodic month. Now have a real good look at the following picture. You should recognize it from the previous—just adjusted a little to help understand what you are about to read.

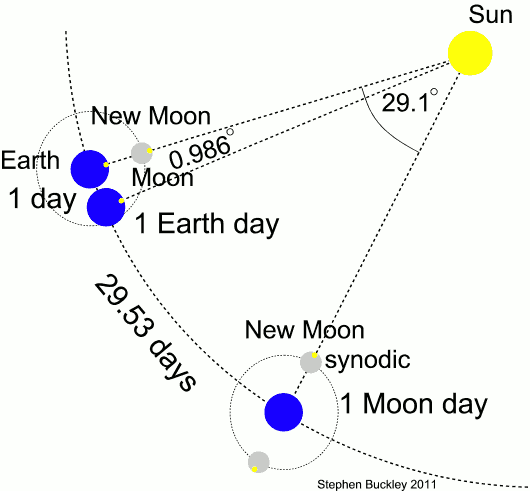
The 1 Earth day at the beginning is included in the 29.53 days. I have put a little yellow spot on the Moon. Now as the Moon orbits the Earth after one synodic month the little yellow spot moves too. Note the extra Moon added for the 3^rd Earth position for the synodic month. This is just showing the Moon at the opposite side of the Earth. This of course would be half way through the synodic month but for comparison showing it here in the 3^rd Earth position. The importance of this is that you can see that the Moon IS actually rotating about its own axis as it orbits the Earth.

Now consider the yellow moon spots on the New Moons. If you were standing on these yellow spots on the moon it would be mid-day. In other words the New Moon to New Moon is mid-day to mid-day for the yellow spot position. What this means is that the New Moon to New Moon has covered EXACTLY one Moon day. What we call the synodic month is no more than the day for the Moon. The synodic month IS one Moon Day.

Now the next bit is a little tricky. When I first thought this out I had to admit I didn't see this one coming.

Here it is. Look carefully at the Moon positions for the New Moon with the yellow spot marking mid-day to mid-day. That's one Moon Day. It's equivalent to 29.53 [approximately] Earth days. For the Moon, it has had to rotate an extra 29.1 degrees to get there. For one Moon Day the Moon has to rotate 360 + 29.1 = 389.1 degrees on its own axis.

Now someone's going to say, No way! That's crazy! One Earth Day is one rotation of the Earth about its axis. So a day for the Moon has to be the same. Guess what. That's wrong too. Have a real close look at that picture again. See the yellow spot I put on the Earth. For mid-day to mid-day on the Earth. For one Earth day the Earth has moved approximately 0.986 degrees in its orbit around the Sun. For the yellow spot on the Earth to get to the next mid-day the Earth has to rotate that extra 0.986 degrees. This means that for one Earth Day the Earth has to rotate approximately 360.986 degrees!! Think about this for minute or two. It takes a bit of getting used to!

The angle value was found as calculated before, but for 1 day we obtain 1/365.25 * 360 degrees and gives approximately 0.985626... or approx 0.986 degrees.

So what's the point of all this?

1. you can be quite comfortable with One Moon Day meaning the Moon has to rotate 389.1 degrees about its axis.
2. The lunar synodic month IS one moon day! As such the construction for a Bible day according to the Lord CAN be used for the Moon to construct the Bible Month since it is also simply the Bible Day for the Moon!

Before continuing, more recently I have found further support from the Bible for the month starting at the beginning of the dark phase of the moon. In Psalms chapter 81 we read:

Blow the trumpet at the new moon, at the full moon, on our feast day.
Psalm 81:3 RSV.
Blow the horn at the new moon, at the full moon for our feast-day.
Psalm 81:4 Jewish Publication Society Bible 1917.
Sound the shofar at Rosh-Hodesh and at full moon for the pilgrim feast,
Psalm 81:3 Complete Jewish Bible 1998.
Blow the horn on the new moon, on the full moon for our feast day.
Psalm 81:4 The Jewish Study Bible, TANAKH Translation, 1999.

There is mention of the new moon and the full moon in this verse. Though this may be hotly debated I believe there is little doubt that this verse shows some form of Bible merism. This is a figure of speech that is commonly used in the Bible. Some definitions follow:

13. Merism: the use of two opposite statements to signify the whole; e.g., day and night, springtime and harvest, hell and high water (Bullinger, p. 435). Note that Bullinger lists these passages under synecdoche, for merism is a kind of synecdoche.  But we shall use a separate category.
“You know when I sit down and when I get up.” (Ps. 139:2)
http://www.christianleadershipcenter.org/616/fospeech.htm
See Quote Note 20.

(literature, rhetoric) Referring to something by its polar extremes.
http://en.wiktionary.org/wiki/merism
See Quote Note 21.

containing a pair of oppositional terms commonly thought of as binaries. It is an expression known as a "merism," wherein oppositional terms are jointly employed to form a totality.
The dead sea scrolls and the language of binary opposition: a structuralist/post-structuralist approach.
http://www.thefreelibrary.com/The+dead+sea+scrolls+and+the+language+of+binary+opposition%3A+a...-a0198354451

Now some people disagree with the full moon translation part in this verse. But it is curious that all the Jewish versions quoted above do mention the full moon. If we do accept the full moon part then this means that we have the two extremes in moon phase mentioned in this verse: the new moon and the full moon. And they are polar extremes or opposites.

The full moon is as bright as the moon can get. The new moon according to this verse would be the exact opposite. Like night to day the Biblical new moon according to this verse is when the moon is as dark as possible. It would correspond to the current astronomical new moon phase. Just so this could not be misunderstood, we mean here, the dark moon.

If we take this view then it is unlikely that the first sliver or waxing crescent could be understood to be the Biblical new moon in this verse.

The more general accepted approach stated for the new moon worship day is the waxing crescent. Well this is the approach the Jews claim to take though their calendar is constructed a little differently. Anyway, an alternative approach we are taking here is to work this from the beginning of the dark phase instead using the Bible construct for a day.

The Bible enforces the dark part of the day to come before the light part. And according to this verse in the Psalms it is quite possible that further support is being given to the new moon of the Bible being the dark moon. This is the approach we are going to take and it is a reasonable alternative.

The rest of this discussion will now focus on determining just where we start the beginning of the dark moon phase to place our location for chodesh according to the Bible construct. Simply put, this will give us the correct day [as best as we can find it] for the new moon worship day according to the Bible.

Some Fun Stuff

Now this is where it gets really interesting. According to the Bible model we need to determine the start of the new moon phase. There is not much information on the internet about when the actual phases begin or end. The simplest approach mentioned is to just divide the lunar month into 8 sections. We will look at this a little later. But first some interesting points to ponder. Before proceeding we need to consider a few points:

• we are approximating the orbit of the Earth as approximately circular. Same for the Moon. For you and me and the average person on the street and for what we are studying here and working out this will do just fine.
• the Moon's orbit is curious. Part of the month the Moon is closer and looks larger in the sky. You can investigate this some time on the internet if you are interested. The actual orbit of the Earth about the sun is also really weird. The Earth and Moon act as a two body system that rotates about a center of mass that is near the Earth but between the Earth and the Moon. As such the actual orbit of the Earth about the sun follows a wobbly curve that follows the elliptic orbit. An astronomer who is plotting the trajectory of some deep space object that might impact the planet will need the correct orbital path. For our study this depth will not be necessary.

There is a minor correction to the second point I have found:

the moon does not orbit the exact center of the Earth, but a point on a line between the center of the Earth and the Moon, approximately 1,710 km below the surface of the Earth, where their respective masses balance. This is the point about which the Earth and Moon orbit as they travel around the Sun.
http://en.wikipedia.org/wiki/Center_of_mass#Astronomy
See Quote Note 22.

It's still a wobbly curve.

OK on to the fun stuff.

If we are going to look at when the phases may be changing we need to be aware of the following.

The Moon is approximately 3500 km wide.
The Earth is approximately 12800 km wide.
The center-to-centre distance is approximately 384,000 km.
You usually see nice sketches of the Earth and the Moon and they are usually pretty close. To scale the reality is more like the following:



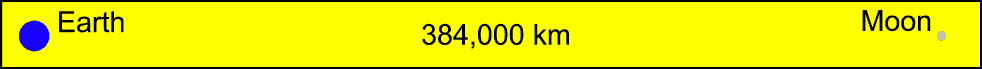
The sizes here and the distance are reasonably to scale. This is it. It's hard to believe from all the nice sketches that are usually drawn. Of course they have to be drawn that way otherwise you just wouldn't see the detail being shown.

The shadow speed on the moon. There are two: on the surface itself, and on the face [we see from Earth].
On the surface the speed is constant. [the following values are of course approximations]
OK correction here—depends on your latitude. The closer to the poles the slower the speed. Use circumference [equatorial] for standard speed.

Moon circumference = 2πr = π × diameter = π × 3500 km = 10995.6 km.
[Wikipedia gives “Circumference 10,921 km (equatorial)”: http://en.wikipedia.org/wiki/Moon]

I used a rough approximation for the diameter so will go with the Wikipedia value.
Equatorial radius 1,738.14 km [Wikipedia]

For 2π radians, 1 rotation = 27.322 days [one siderial month]
so speed = 10921km/27.322 days = 399.71 km/day = 16.65 km/hr

Radial speed: we have 2π rad = circumference
so the radial speed is = 2π rad/27.322 days or



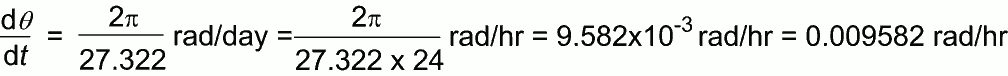
We can check this:
Using

then

So the moon equatorial surface speed = 1738.14 × 0.009582 km/hr = 16.65 km/hr as above.
This value which is approximate is pretty close to the correct value given on Wikipedia of
4.627 m/s.
Converting this to km/hr gives 16.6572.

The problem we are now faced with is, how long is a piece of string?
The Bible model for the month starts at the beginning of the new moon phase. And this can obviously get hotly debated. We are entering the part where the waters get pretty murky.
As one quote we had before stated:

Technically, each phase, just like the one called a New Moon, when the Moon is exactly between Earth and the Sun, lasts only a brief instant. But to our eyes, a New Moon can last for a few days, representing the time that the Moon appears in the sky too near the Sun's position for us to see it at all.
http://spaceplace.nasa.gov/en/kids/phonedrmarc/2004_march.shtml
See Quote Note 1.

How many days is a few days? Well, the following quote is from the U.S. Naval Observatory about the “Crescent Moon Visibility and the Islamic Calendar.” So why are we now interested in the crescent moon? Well when the crescent moon becomes visible this basically marks the visible end of the new moon phase [correctly or incorrectly]. That will help us determine the length of the new moon phase. Here's the quote and it reads pretty dismally:

Crescent Visibility:
The visibility of the lunar crescent as a function of the Moon's "age" - the time counted from New Moon - is obviously of great importance to Muslims. The date and time of each New Moon can be computed exactly (see, for example, Phases of the Moon in Data Services) but the time that the Moon first becomes visible after the New Moon depends on many factors and cannot be predicted with certainty. In the first two days after the New Moon, the young crescent Moon appears very low in the western sky after sunset, and must be viewed through bright twilight. It sets shortly after sunset. The sighting of the lunar crescent within one day of New Moon is usually difficult. The crescent at this time is quite thin, has a low surface brightness, and can easily be lost in the twilight. Generally, the lunar crescent will become visible to suitably-located, experienced observers with good sky conditions about one day after New Moon. However, the time that the crescent actually becomes visible varies quite a bit from one month to another. The record for an early sighting of a lunar crescent, with a telescope, is 12.1 hours after New Moon; for naked-eye sightings, the record is 15.5 hours from New Moon. These are exceptional observations and crescent sightings this early in the lunar month should not be expected as the norm. For Islamic calendar purposes, the sighting must be made with the unaided eye.
http://www.usno.navy.mil/USNO/astronomical-applications/astronomical-information-center/cres-moon-islamic
See Quote Note 23.

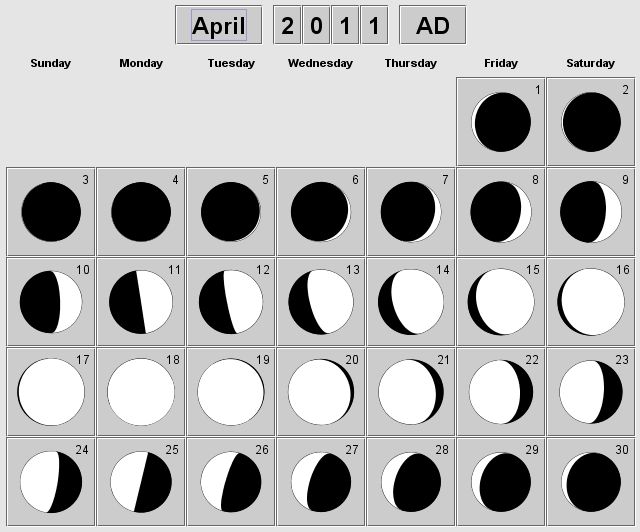
15.5 hrs that's hardly a day. Doubling this gives us 31 hours and not much more than a day. The general internet sites showing pics of the moon on various days also add to this muddle. As follows it looks like:


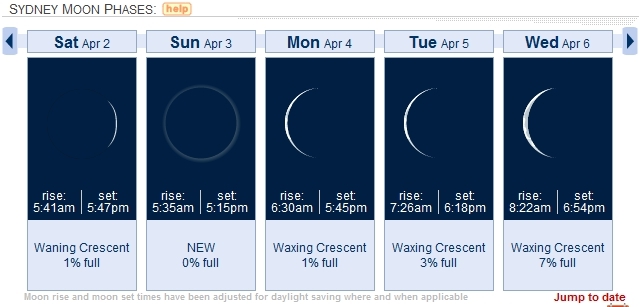
http://moonphases.willyweather.com.au/nsw/sydney/sydney.html


http://paulcarlisle.net/mooncalendar/

http://www.dacre.net/moon/moonphase.html

http://www.dacre.net/moon/moonphase.html

http://www.dacre.net/moon/moonphase.html

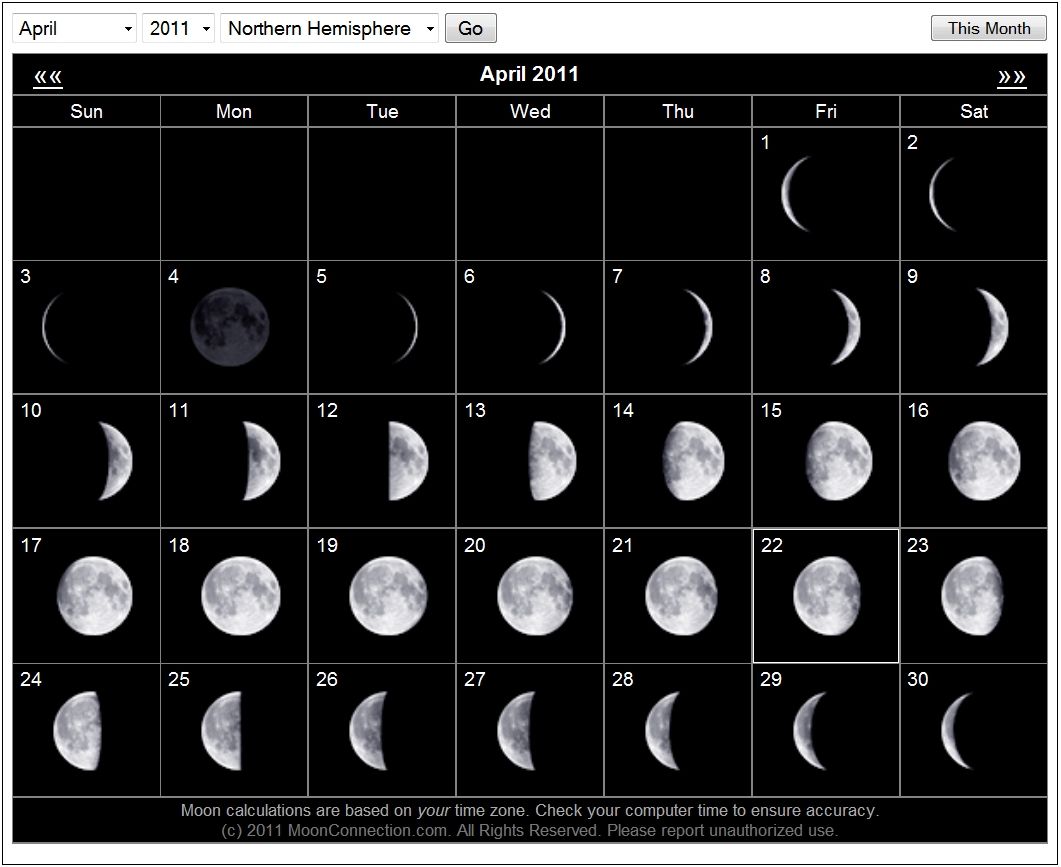
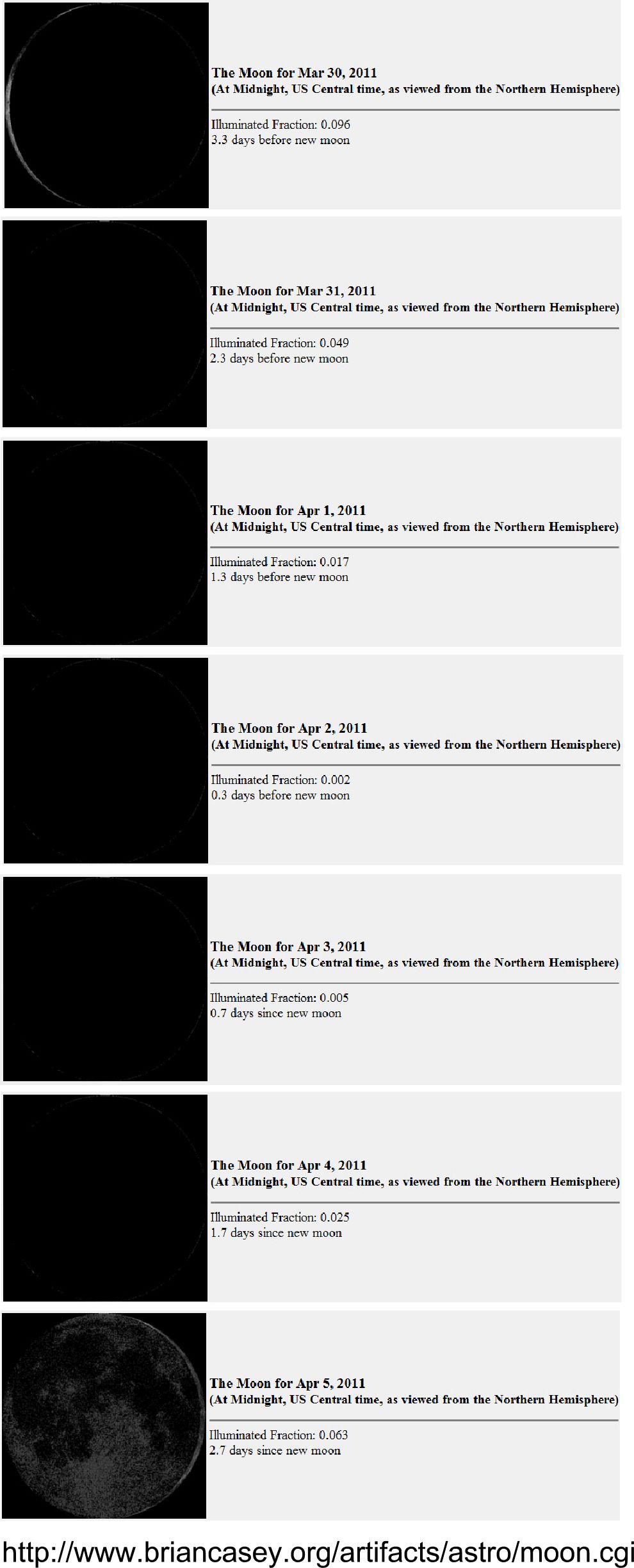
Well looking at all these site pics showing phases of the moon about the astronomical new moon date the length of the new moon phase could be anywhere from 1 to 6 days.

2 of them show 1 day for the phase.
1 shows 2 days.
1 shows 3 days.
1 shows 5 days.
and
1 shows 6 days!

Of course, all this is a little loose. As the pics were each generated for just one time of the day, noon or midnight or whatever. Saying that, there is clearly some leeway as the new moon could actually extend into the next day up to about noon [or midnight etc]. So these figures could all be extended by one day! That would give anywhere from 2 to 7 days for the length of the new moon phase! Is there any way to sort this out??

A numerical division may not give the exact answer but it could be a good guide just what direction to move in. Simply put, lets take the time period for the month and divide by the number of phases.

We have 8 phases generally accepted: New Moon, Waxing Crescent, First Quarter, Waxing Gibbous, Full Moon, Waning Gibbous, Third or Last Quarter, Waning Crescent, and then a New Moon again.

This would give

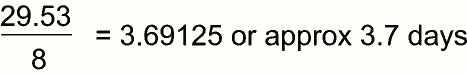
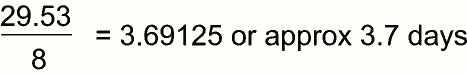
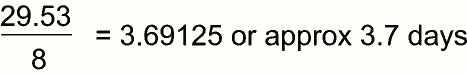
Halving this amount would give 1.85 days either side of the astronomical New Moon time. It's probably reasonable but this result was obtained using a linear approximation to divide the phases. The problem is, as we see them on the face of the Moon, the phases are not linear. Here's why. We worked out before that the radial speed of the Moon about its axis is about 0.009582 rad/hr.

This translates to the equatorial surface speed approximately at 16.65 km/hr.

Is this the same as the shadow speed across the Moon's surface? Apparently not. The reason for this is that all the phases occur in the synodic month of 29.53 days. The equatorial surface speed was calculated using the sidereal month of 27.3216 days.

Why the difference then? Basically these are different because the Moon is also orbiting the Earth and it's just not a simple rotation about its axis. Let's consider a diagram of the Moon with the shadow line rotating. The Moon is also rotating but for this diagram we will ignore that. Also given that we will determine an equatorial speed in km/hr we still won't really see this speed correctly because of the curvature of the Moon. When we look at the Moon it is so far away it is like looking at a flat disc. The following diagram shows this better:

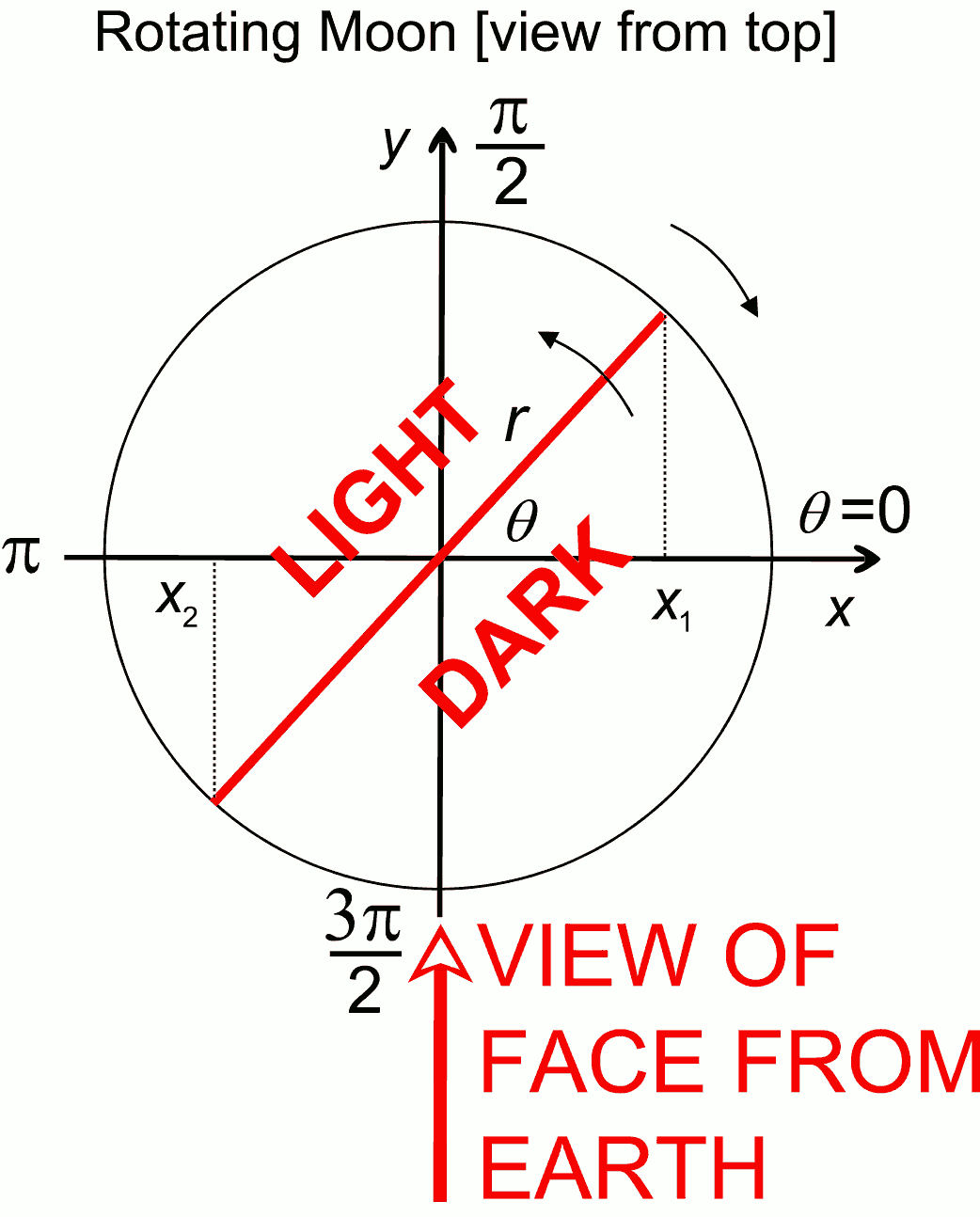
This is the view from the Southern Hemisphere. The Moon is rotating clockwise and the line of shadow/light appears to rotate anti-clockwise. From the Southern Hemisphere the line dividing the dark and light parts of the face of the Moon travels from left to right. As θ travels from zero to 2π we see that the full cycle of phases is taken:

θ = 0 New Moon
θ = π/2 First Quarter Moon
θ = π Full Moon
θ = 3π/2 Last Quarter Moon
θ = 2π New Moon again and is the same position for θ = 0.

Note that during this same period of time the Moon has rotated 389.1 degrees about its own axis which is more than 2π radians.

As the shadow line rotates we see the shadow/light position on the face denoted as x₁ and x₂ [depending which division line we see] move forwards across the face of the Moon.

Note: x₂ = −x₁ but for simplicity in the diagram above labelled it as x₂.

We can safely assume that the angle theta is increasing approximately at a constant rate. But as it passes through π and zero again, the point x₁ [or x₂] will appear to slow in movement.

Note that as θ passes through zero the shadow line will move out of view behind the face of the Moon. As this happens the opposite end of the diameter shadow line will appear at the other extreme. So as point x₁ disappears from view x₂ appears at the other extreme and moves across the face of the Moon. And the process repeats. You see this as the Moon moves through the New Moon phase. The shadow line shows a waning crescent at one side and then disappears. And then moving through the New Moon phase the next thing you see is a waxing crescent appear at the OPPOSITE side of the face.

As the shadow line gets to the extreme edges the speed will actually drop to zero instantaneously and then increase again with maximum speeds attained at π/2 and 3π/2. These are the radial positions for the First and Last Quarters. The slowest speeds [of zero km/hr] will be attained at the instantaneous points of the New and Full Moons. The mathematics of this gives us an amazing result. The longest phases are the New and Full Moons. All the other phases are shorter!

We can determine the formula for x₁ in terms of θ mathematically as

where r is the radius of the Moon. A corresponding velocity formula for x₁ as it moves across the face of the Moon can be found as:

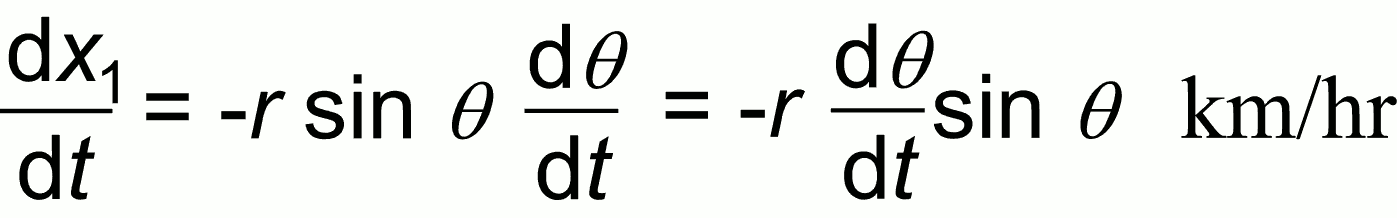
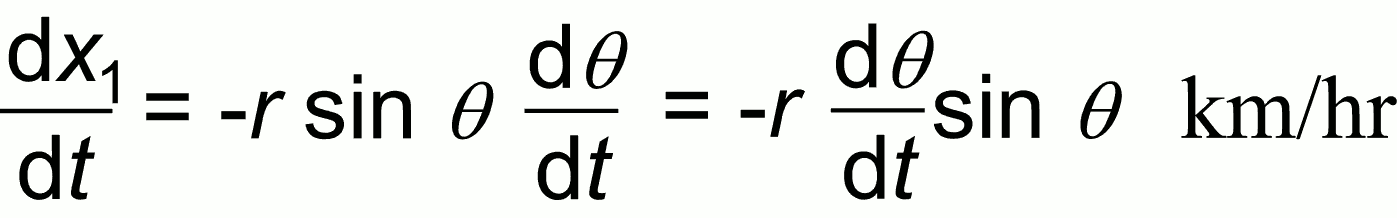
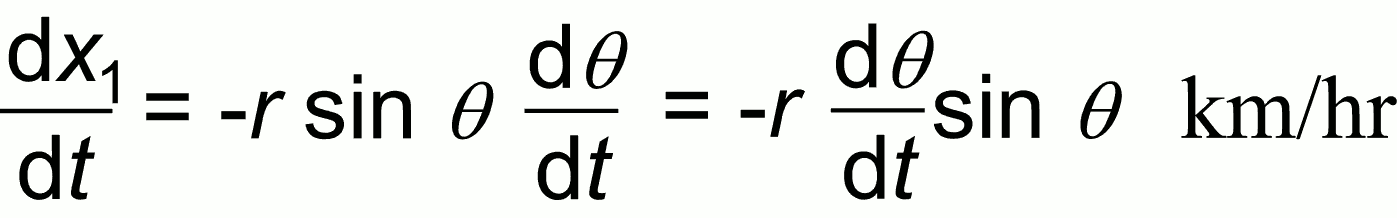
The radial rate of change here is different to the radial velocity of the Moon. This is because this calculation is independent of how fast the Moon is rotating itself about its axis. We simply have the phases during the synodic month of 29.53 days. That's it. Having said that, we can work out the value in km/hrs as follows:

θ travels 2π radians in 29.53 days so that gives



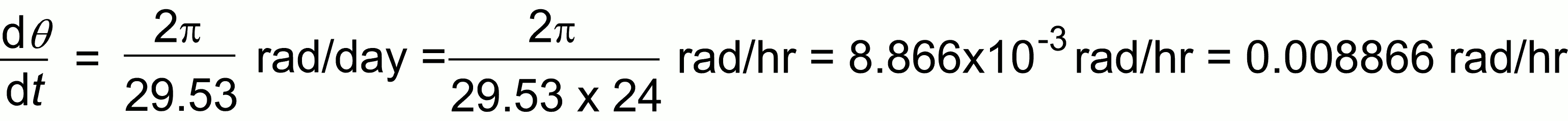
Using l = rθ then the equatorial shadow speed can be found as:

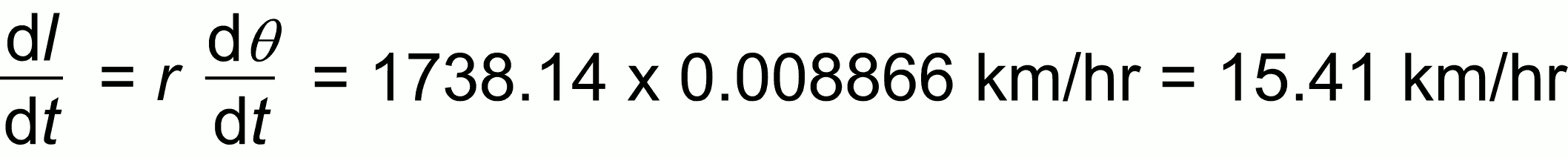
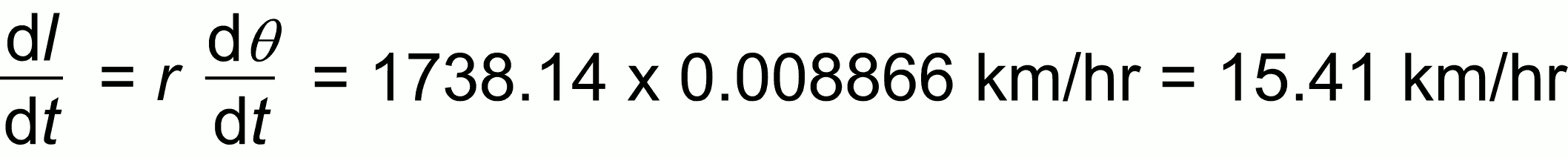
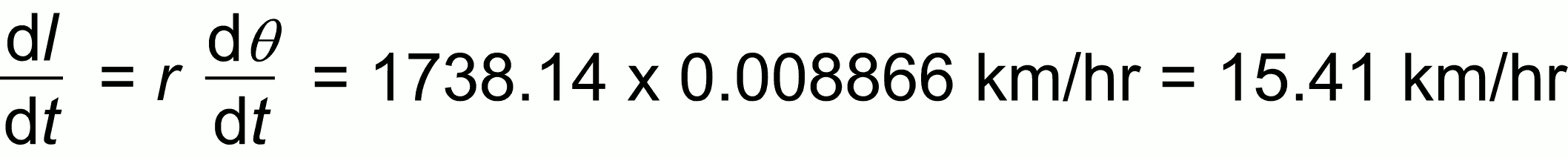
This is on the surface of the Moon. To adjust this for the face of the Moon we require the previous formula:

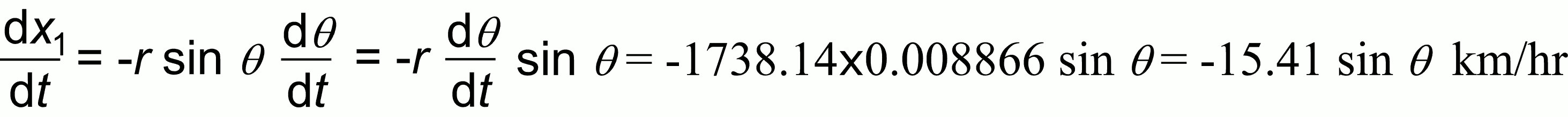
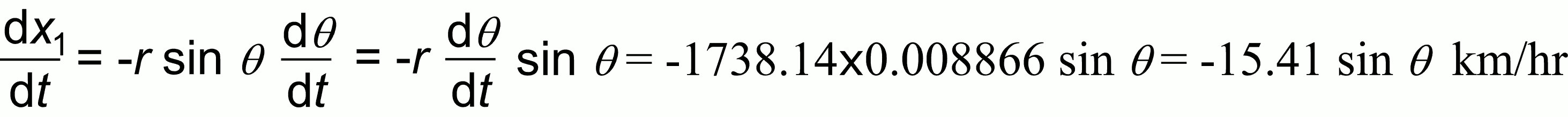
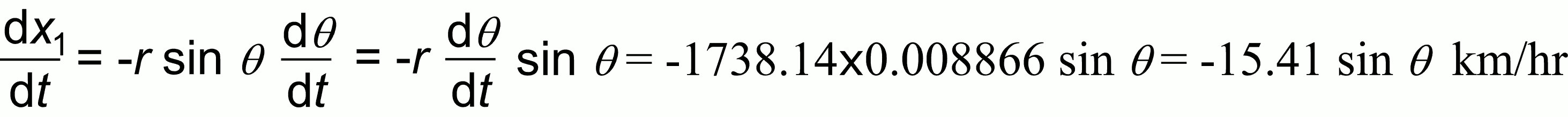
The equatorial shadow speed we worked out was 15.41 km/hr.

The last formula will obtain this value in magnitude for θ = π/2 and 3π/2 at the First and Third Quarter Moons when the shadow is closest to us and moving the fastest. And at the extremes corresponding to the astronomical New Moon and Full Moon the speed drops instantaneously to zero as mentioned before.

This equatorial shadow speed formula was important as it tells us that the shadow is moving the fastest at the First and Last Quarter Moons and slowest at the New and Full Moon phases. This means that the New and Full Moon phases are longer than the other phases. The lengths of the phases is not constant. Perhaps we didn't really need this shadow speed formula but it was important to know how the position x₁ moves as the shadow line moves with a constant radial velocity.

It's not linear so 3.7 days for each phase won't work. What will? As stated before we see the face of the Moon more as a flat disc from the Earth. Dividing up the phases may be simply a linear division along the horizontal diameter. We will show this approach is actually reasonable but first let's try the calculation. The formula we have is:

but we can simply work this for a unit circle with r = 1 and obtain identical results. In one run of x₁ for θ from π to 2π this translates to x₁ from −1 to 1. We have Full Moon, Waning Gibbous, Last Quarter, Waning Crescent, and finally New Moon. The Full and New Moon phases are at the extremes so we only have half of these. This gives:

0.5 Full Moon + Waning Gibbous + Last Quarter + Waning Crescent + 0.5 New Moon = 4 full phases. The unit circle diameter length is represented by 2 units. So 2/4 = 0.5. Each phase is represented by 0.5 units. The positions of interest are as follows. The first position is the end of the Full Moon phase at:

−1 + 0.25 = −0.75

then the end of the Waning Gibbous phase should be −0.75 + 0.5 = −0.25

the end of the Last Quarter Moon phase should be −0.25 + 0.5 = 0.25 and finally the end of the Waning Crescent phase should be 0.25 + 0.5 = 0.75

Working out the first angle for the half Full Moon position gives

cos⁻¹(−0.75) = 3.8643 rad [in the third quadrant]

The angle then contained in the half of the Full Moon period is 3.8643 − π ≈ 0.723 rad or approximately 3.4 days and should be the same for the New Moon phase. We don't have to work out any other values as this gives the New Moon phase length to be approximately 6.8 days. This is probably far too high and the Waning and Waxing Crescents will most likely be seen too far into this phase for this number to be of any use. It is, however, interesting to note that one of the values determined from the web sites showing Moon phases did allow for a length of 7 days. In general though this does sound a little high.

The next approach was to determine, if possible, a mathematical formula for the percentage illumination of the face of the Moon. This may be a little more helpful than the last approach with the angles though the two approaches are actually related. It turned out that this formula was extremely helpful in determining a upper bound [more about this later].

So what are we doing? You look at the face of the moon and there is some part in light and the rest dark. What is the percentage of the moon face that is illuminated?

Basically we have something that looks like:

It may actually be difficult to determine the correct shape of the curve to get this exactly so some simple model approximations were considered as follows [though this was resolved using spherical coordinate geometry]. The models I investigated were rectangular, trapezoidal, parabolic, and exact using spherical coordinate geometry. They all yielded the same formula solution.

To simplify the mathematics we will consider the model dimensions about 1 or similar for calculating ratios. Further only the simplest model is covered here. It should be pretty easy for anyone to follow. If anyone is keen to see the other working time permitting I will place them on a separate page.

Rectangular Model:

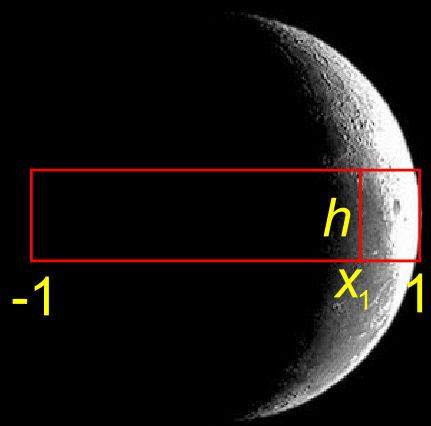
Remember we are after a percentage of illumination. This is like a ratio so the actual correct area is not needed. A simple approximation should suffice.

The light rectangular area is (1 − x₁)h ; the total rectangular area is 2h. Then the percentage illumination is approximately:

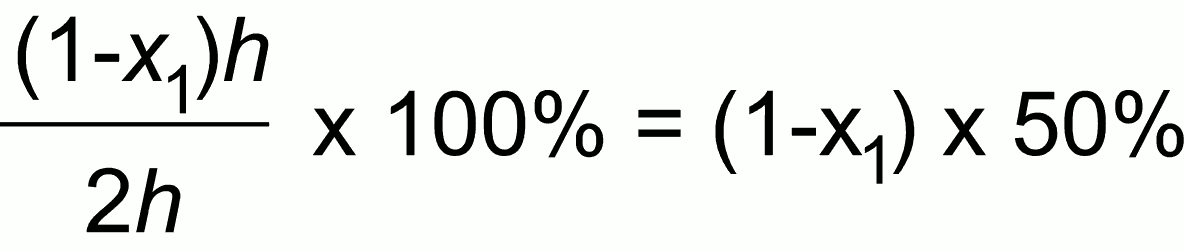
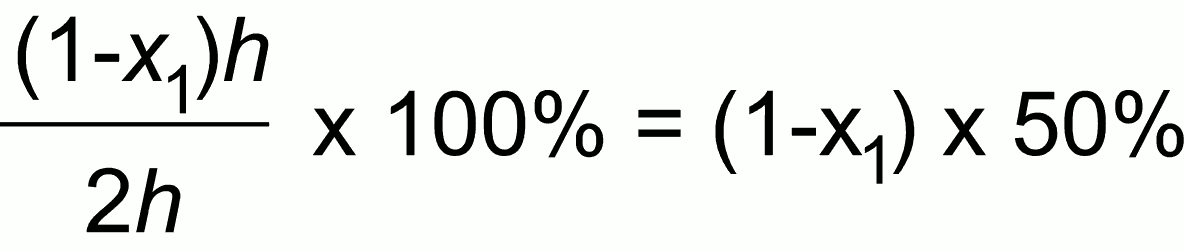
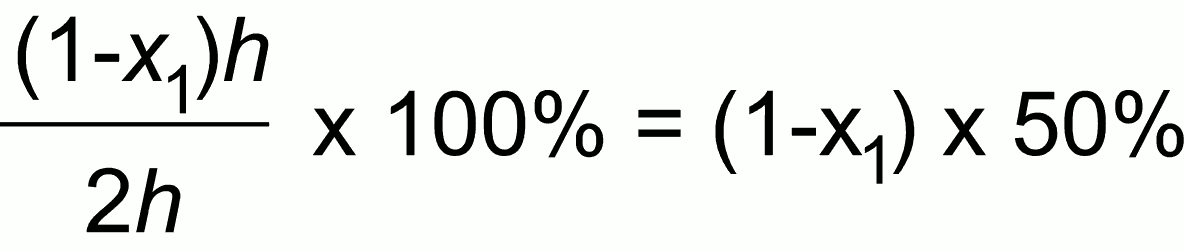
For our purposes this formula works pretty well. The other mathematical models all gave the same formula.

This formula will be used shortly and we will find it very helpful.

Now the question. Can we make any sense of all of this?

A working solution

First the visual sighting approach. I have tried looking at a few sunset times to see if I could notice the new moon—either view; first sliver of crescent or dark moon and have to admit not being successful with either. The crescent [waxing or waning] was more noticeable the further from the astronomical new moon time I looked, as expected. But what about the location of the moon to the sun? The astronomical time is dependent on the moon sun positioning, not sunset times. Simply put, some new moon times [astronomical] are given for during the night—depending on your location. But you can't see the new moon during the night as the moon sets and rises with the sun. Similarly the event could occur at midday and again a sunset reading would be hours away.

A simple solution to this problem would be to determine some approximate numerical value that could be subtracted, or added to the astronomical new moon time according to whichever “new moon” you were using. The ancient Israelites were far from stupid and it would not have taken them too many years to work out the approximate value for the synodic month [if they already didn't know it] and the fact that the periods follow a sinusoidal pattern through the year. This information could have been easily used to determine the approximate date of the next new moon at any time of the year. Of course where we are now we readily have available astronomical new moon times so don't need a sinusoidal chart, just a numerical value to find approximate new moon dates. By new moon dates here we mean either the Jewish new moon or the Bible model.

Also since the sighting approach is all approximate this is not really an exact science so as an alternative we don't really need to have a number to 10 decimal places of precision. A rough approximation should suffice. With the information we have at hand it is actually possible to determine a rough approximate value. Further the value we obtain appears to hold reasonably well with the Jewish calendar dates. Also using the Jewish calendar it is possible to make a correction in the value, if we want to use it.

So let's see how all this works...

We can easily obtain a lower value by looking at the data from the internet sites for [astronomical] new moon periods. From before we obtained:

2 of them show 1 day for the phase.
1 shows 2 days.
1 shows 3 days.
1 shows 5 days.
and
1 shows 6 days!

But allowing for the reading extending half a day in either direction extends these periods by one day giving:

2, 3, 4, 6, and 7 days displaying as possible periods.
We can be sure of one thing—they all agree with a minimum value of 2 days for the period. Taking this from a central point we obtain a lower value of 1.0 days from the astronomical new moon time. This is where we start. Now how do we obtain a maximum possible value? That's not too hard either. Remember the picture of the new moon on the Jewish site? The following shows this rotated.

I rotated this again and took some measurements as follows:

By drawing a line and measuring this approximately I obtained a value of 0.826 on the right hand side where the shadow changes to light. This is probably a bit rough but should be reasonable for what we are doing here.

The percentage illumination can be found using the formula we obtained to give

Illumination = (1 − 0.826) × 50% ≈ 8.7%

and using cosθ = x₁ = 0.826 we find θ ≈ 0.5988 rad and from this we get approximately 2.8 days.

For 8.7% illumination the light part looks pretty obvious so I would suggest dropping this. Trying for simple numbers I would suggest dropping 2.8 down to 2 days, as 2.5 probably would still be showing a fair section illuminated which would be visible.

We could also look at the pic obtained from the other Jewish site mentioning the new moon. Again a rotated version gives:

Doing an approximate measurement on this we obtain:

The value obtained here is approximately 0.906.
The approximate illumination can be found using the formula as follows:

Illumination = (1 − 0.906) × 50% ≈ 4.7%

and using cosθ = x₁ = 0.906 we find θ ≈ 0.437 rad and from this we get approximately 2.05 days.
That is, this view of the moon is at approximately 2.05 days from the astronomical new moon time.

As before, we could definitely set 2.0 days as a top bound for an approximate numerical value.

So what do we have?

We have a lower value of 1.0 days.

We have an upper value of 2.0 days.

It should be pretty obvious just where this is going.

The answer is clearly between these two numbers. Somewhere. What's the simplest value we could try that would be very easy to use. It's not really hard to guess this.

Mathematically there is a numerical method called the bisection method that is used when you have two initial guesses as the solution to a problem. The next guess is simply the average point. In this case we would obtain 1.5 days and this seems like a good point to start. It may even do completely.

Let's just see how a value of 1.5 days works. Can we test this? Yes we can by using the value forwards and see how it compares with the Jewish new moon dates.

We will use this value for determining Jewish Rosh Chodesh dates for 2011. The first astronomical new moon date is 4 Jan 11.03am. We are using here, of course, Jerusalem times. Moving forwards 1.5 days is very easy: just add 1 to the day and add 12 hours—simple by changing am/pm to pm/am. If the original time is am it just moves to pm. But if the original time is pm you move to am but for the next day so add 1 to the date.

For 4 Jan 11.03am 1 day takes us to 5 Jan 11.03am. Then adding 12 hrs we get to 5 Jan 11.03pm.

The final step in this process is to compare this time with the sunset time. Obviously this really only needs to be done if you are likely to be anywhere near it. At 11.03pm at night this is not likely to be the case. However, it is late at night and obviously AFTER sunset of 5 January. The Bible reckoning for a day is from sunset to sunset. This is how the Jews keep the day and why they keep sabbath from Friday sunset to Saturday sunset. Since the time we have arrived at is AFTER sunset we are really in the next Jewish day. Then we take chodesh as the NEXT day AFTER January 5. We then arrive at 6 January for chodesh.

The Jewish calendar for January 2011 gives Rosh Chodesh on 6 January.

This process was continued with all the astronomical new moon dates in 2011 and every one of them landed on a Jewish new moon or Rosh Chodesh day using the numerical value of 1.5 days. Sometimes the Jewish calendar had 2 Rosh Chodesh dates in the month. Each time this happened the 1.5 day calculation landed on one of them. For 2011 in EVERY case the method was completely successful in landing on a Jewish Rosh Chodesh date.

The next question to ask was, just how well did the numerical value of 1.5 days fit the Jewish calendar? There appeared to be a reasonable amount of flexibility especially when the Jewish calendar gave 2 Rosh Chodesh dates. The main points of interest then fell on the single day calculations. For example, April gave a range of −10.32 hours to +13.29 hours flexibility in the 1.5 day numerical value. This is explained in a bit more detail as follows.
See insert for 2022 below.

The Jerusalem astronomical new moon time for April is given as 3 Apr 5.32pm. Adding 1 day takes us to 4 Apr 5.32pm. Now adding 12 hrs we get to 5 Apr 5.32am. Note we moved to am but the next morning so the date moves to 5 April.

With the numerical value of 1.5 days we have landed at 5.32am. We take chodesh as 5 April. The question we are asking is how much variability from 1.5 could we take and still stay in 5 April as chodesh? To answer this properly requires the sunset times at both ends of the Jewish day. So we need the previous sunset and the sunset for 5 April. For Jerusalem these were found as 7.00pm and 7.01pm. From 7.00pm the previous night we have 5 hours to midnight and to 5.32am we have 5 + 5.32 or 10.32 hours or 10 hours 32 minutes. So in theory the value of 1.5 days could be reduced nearly 10 hours 32 minutes and still give a Rosh Chodesh date of 5 April. Similarly at the other end of the day we obtain 7.01pm or 1901 − 0532 giving 1329 or 13 hours 29 minutes we could extend the 1.5 numerical value and still obtain a Rosh Chodesh date of 5 April.

The date for April shows that the 1.5 numerical value is nearly in the middle or centrally located for these extremes. However, trying some other dates we may be able to tighten the boundaries a bit. This is indeed the case and an interesting adjustment can be obtained using February and November.

The Jerusalem astronomical new moon time for February is 3 Feb 4.31am. Adding 1.5 days takes us to 5 Feb 4.31pm.* Sunset is 5.17pm so we take chodesh as 5 Feb. This is one of the months the Jewish calendar gives 2 Rosh Chodesh dates: 4 and 5 Feb. However we only have leeway of 46 minutes to sunset. So we have a right hand bound on the numerical value of 0.46.
* See insert for 2022 below.

Similarly for November we start at 25 Nov 8.10am. Adding 1.5 days takes us to 26 Nov 8.10pm. The Jerusalem sunset time for 26 Nov is 4.36pm. Since we have landed at 8.10pm this is well after sunset and we choose the next day 27 November for chodesh. Further this only gives a leeway of 8.10 − 4.36 = 3.34 or 3 hours and 34 mins.

So to fit the Jewish calendar the numerical value of 1.5 days is squeezed on the left by 3 hrs 34mins and on the right by 46 mins. A simple adjustment for the Jewish calendar would be to have our numerical estimate for chodesh centrally located between these two extremes. This adjustment can be easily determined by taking the average of the values. −3.34 + 0.46 gives −2.48 and then divide by two to obtain −1.24 or approximately minus 1 and a half hours. [the decimal parts in these numbers are minutes so 0.60 would become 1.00, that is 60 mins = 1 hr].

The adjustment in our numerical approximation would be to subtract 1 hr 30 mins. That's it. It is not really much at all. The value of 1.5 days was a very good estimate. We could nearly leave it as it is. But an adjustment of 1 hr 30 mins is not hard to do.

For an approximate value for finding the Jewish chodesh dates the adjustment would be to subtract 1 hr 30 mins from the numerical estimate of 1.5 days giving 1 day 10 hrs and 30 mins.

What about the Bible model we have proposed? Do we subtract or add this adjustment? The numerical value in the Bible model is for the reverse direction from the astronomical new moon time. But basically as we travel through the phases of the moon we are moving in a positive direction from the waning crescent to the new moon and then the waxing crescent. Approximate numerical values in time are suggested for these two points that would be in reasonable accord with visual sightings. An adjustment has been proposed which would have us move the numerical value for the waxing crescent phase back one and a half hours. This suggests that the previous phase approximation could also be out and should be moved back the same amount of time.

However, some may argue by symmetry it should be subtracted. Unfortunately this is a pretty good point. But from the sample of new moon pictures obtained from internet sites giving possible ranges of the phase from 2 days to 7 days I would be very hesitant to subtract any value from our numerical approximation. It is an approximation. It does actually work well for the Jewish dates even without the adjustment. That the Jewish calendar dates suggest an adjustment of only 1 and a half hours is pretty good evidence that the approximation is pretty close to where it should be. As such for the simplicity of the calculations I propose that the numerical value of 1 and a half days be left where it is for the Bible model. Simply put, it's close enough.

This brings us to a final question.

One Rosh Chodesh day or two?

This may seem a silly question but it must be noted that when you look at the Jewish calendar just about every couple of months they have 2 Rosh Chodesh days listed next to each other. Some of the previous Jewish quotes we looked at mentioned this:

5. The Hebrew New Month, as it goes according to this calculation is either on the 30th or 31st day each month, as a month is 29.5 days, we have alternating New Months, being the 30th (and) or 31st day.
6. So one month the New Month is celebrated on the 30th day, and the month after it is (usually) celebrated on the 30th and 31st day.
Reply from colelchabad.

Sometimes Rosh Chodesh is one day, but sometimes it is celebrated for two days. Months are based on the lunar cycle, of course. The amount of time required for the moon to make one complete revolution around the earth is determined by the conjunction of the earth, moon, and sun (i.e. they lie along a line). When this happens, it is the new moon. A complete revolution takes 29 days, 12 hours, 44 minutes, 3-1/2 seconds. Days in our calendar must begin at sundown, regardless of when the conjunction actually takes place. Hence it is necessary to either add or subtract a half a day from each calendar month. For this reason, Hebrew months alternate between 29 and 30 days in length, with the actually moment of the new moon falling in between.
Source: Rabbi Scheinerman's homepage
http://www.jewishvirtuallibrary.org/jsource/Judaism/Rosh_Chodesh.html
See Quote Note 2.

As noted before there is a simple error in this quote—the period of time for the Moon to make one revolution about the Earth is the siderial month of 27.32 days, not the synodic month which is actually wanted here. A simple mistake. But we have the reasons given for the 2 days. The synodic month is of length 29.5 days approximately. So one month it is celebrated on the 30^th day and the next on the 31^st. But to be sure they don't get it wrong for the second one they do both: the 30^th and 31^st days. An interesting solution. Should we be interested in doing this too?

The best way to investigate this is to try our numerical solution for the Jewish calendar for 2011 and see just how the Rosh Chodesh days fall with the numerical solution. We of course will use the Jewish calendar correction for 2011: 1 day 10 hours and 30 mins. And for the Jewish calendar dates we will use Jerusalem times. The following table will list the astronomical new moon dates and times, the numerical solution with the adjustment for the Jewish calendar, the sunset time for Jerusalem, the selected date for chodesh, and the actual Rosh Chodesh dates given in the Jewish calendar. We then obtain:


Note: if you want to see the values obtained previously for 1.5 days just add 1 hr 30 min to the Numerical column values.
Also the Jewish days for September 2011 are called Rosh Hashanah--this is the name the new moon day is given when it falls on the beginning of the Jewish year.

This table raises more problems than it solves. I was expecting that the numerical times for the 2 Rosh Chodesh dates would be near the sunset times. This actually does appear to be correct for a majority of the months: February, May, July, and August. The last three fit well with the numerical value near the sunset time in the middle. But February is near the edge of the second day. The adjusted numerical value as expected appears to be centrally located. The extremes of February and November give numerical times approximately 2 hours near sunset at both ends. For February another few hours will take us to another day for Chodesh. Similary for November a few hours back will force us to the previous day for Chodesh.

There appears to be some issues with the workings for the months with 2 Rosh Chodesh dates. According to the Jewish quotes this should happen every couple of months as they adjust for the half a day in the lunar month. But this calendar shows 2 days for the months of February and March consecutively and then again three months in a row later in the year: August, September, and October.

The answer to this puzzle is that perhaps the Jewish calendar is using exactly 29.53 days to go from one lunar month to the next. The value of 29.53 days is an approximate average value. The periods for the actual synodic months vary through 2011 with a maximum value of about 29.76 attained in the first half of the year and a minimum value of 29.34 through the second half. This is reasonably obvious from the table constructed above. For the first half of the year the approximation of 29.53 days is lagging behind the actual synodic month so the placement of the two days in February and May are showing an earlier position. The numerical value lands on the second of the dates. This doesn't appear to be the case for March but I believe that that is most likely because March shouldn't actually have 2 days anyway. Similarly for all the 2-date months in the second half of the year, EVERY one has the numerical solution landing early on the first day as the true synodic month is being followed and lagging with the Jewish calendar.

The numerical solution was found so as to follow the actual time of the astronomical new moon. As such, it should follow the actual synodic month more closely as the period lengths change through the year. It's not perfect and perhaps even needs adjustment as the lengths shorten and get longer. But even as it stands, for 2011 at least, it should follow the actual synodic months closer than just using 29.53 days continually between each month. Having said that, I have no hesitation whatsoever saying that some of the Rosh Chodesh dates given in the Jewish calendar for 2011 are questionable. Mainly Feb 4, Mar 7, May 4, Jul 2, Sep 30, Oct 29, and Dec 27.
Note: I adjusted my thinking on this and later accepted May 4 and Jul 2 as reasonable.

OK this is now official. I have been able to obtain actual Molad times for Jerusalem. From a table I have extracted 4 Molad dates:

3 Feb 11.16am
5 Mar 0.00am [listed as 12.00am I would prefer to write this as 0.00am so there is no confusion].
29 Aug 4.24am
27 Sep 5.08pm
http://www.chabad.org/library/article_cdo/aid/216238/jewish/Molad-Times.htm#notes
See Quote Note 25.

From some correspondence I received earlier [not fully quoted] the comment was given:

We have a New Moon every 29 days, 12 hours and 44 1/18 minutes, so once you have the new moon for any given month you can figure every future one by adding the 29.12.44.1/8.
4. The last New Moon was last Friday 12:00.07 (just past midnight) or 12 plus 7/18 of a minute, so the next one will be Sunday afternoon at 12:44 and 8/18 minutes.

The correspondence date was Thursday March 10. The last Friday referred to here would then be 5 March as given above. The Molad table gives the time as 12.00am so is the same time in the correspondence of 12:00.07 just past midnight. Note the quote calls this the New Moon. This is supposedly the exact “astronomical” time of the Jewish new moon according to this quote.

We now call this into serious question. Note the 4 Molad date and times I have listed above. From the February to March date time we obtain doing this as a subtraction 29 days 12 hours and 44 minutes. Similarly for the August to September date and time we would also obtain by subtraction a value of 29 days 12 hours and 44 minutes.

That is, the the next molad date and time has been obtained from the previous month by adding exactly 29 days 12 hours and 44 minutes.

So what's wrong with that? I picked these 4 months specifically for a reason. The first two months of February to March give the longest astronomical length in the synodic month for 2011. Approximately 29.76 days or 29 days 18 hours and 15 minutes.

Similarly for the last two months of August and September, these actually give the shortest astronomical length for the synodic month in 2011: Approximately 29.34 days or 29 days 8 hours and 5 minutes.

So the Jewish calendar is clearly not following the lunar synodic months correctly through the year. The molad times as given in these tables sadly would most likely be just about all wrong.

JUDAISM 101
The Jewish Calendar: A Closer Look
Note that the calculated molad does not necessarily correspond precisely to the astronomical new moon. The length of time from one astronomical new moon to the next varies somewhat because of the eccentric orbits of the Earth and Moon; however, the moladot of Rabbi Hillel's calendar are set using a fixed average length of time: 29 days, 12 hours, and 793 "parts" (or in Hebrew, chalakim). The amount of time is commonly written in an abbreviated form: 29d 12h 793p.
http://www.jewfaq.org/calendr2.htm

Considering this, the next few paragraphs could most likely be jumped, but I will leave them in for historical interest. Stephen.

This actually does sound pretty bad and any Jews reading this are most likely to be disheartened. But on the good side most of the Rosh Chodesh dates given in the 2011 calendar do appear to be reasonable. This is good news.

I would propose a simple solution to this problem. Pretty much what I have worked out in this search. Adding some numerical value to the astronomical new moon time to arrive at the correct date for Chodesh. It certainly couldn't do worse than using a table of values that are mostly incorrrect as the length of the synodic month changes through the year. And having a numerical value linked to the astronomical new moon time will ensure that as the month length changes the dates for Rosh Chodesh will follow accordingly.

Will this work for the months with 2 Rosh Chodesh dates? Yes. I have given this some thought and believe that the Jewish approach of 2 Rosh Chodesh dates does have some merit. Specifically when the calculation falls just before and near a sunset time. Both days could be called.

I have also given this some further thought and finally decided that the Jewish approach may be safer when the time falls about half way or close to the sunset time either before or after. This will add a few extra days to the original proposed chart: 4 May, 2 Jul, and 26 Nov.

Taking this approach a proposed table for 2011 would then be as follows:



Note that for the middle of the year we find the numerical model gives the days exactly the same as the Jewish calendar. But as we move away from the middle of the year the synodic month lengths change and some discrepancies appear in the dates chosen.

Closing thoughts

This approach is pretty simple but some care may be needed. It could be argued that the numerical value is for a standard month which may be the 29.53 days. This could be investigated just which length this was optimal for. Having determined the correct length [which hopefully should be for 29.53 days] it could be further investigated whether the number needed any adjustment as the month lengths changed since it is tied to a fixed length. The adjustment most likely should be minimal and may not be necessary. However, note the following:

Because of perturbations in the orbits of the Earth and Moon, the actual time between lunations may range from about 29.18 to about 29.93 days. The long-term average duration is 29.530589 days (29 d 12 h 44 min 2.9 s).
http://en.wikipedia.org/wiki/Month#Synodic_month
See Quote Note 26.

This gives a worst possible range of 0.75 days or 18 hours. This may be extreme and for 2011 only about a 10 hour range occurred. Still this may mean that a close watch on the synodic month lengths should be given each year.

End of the walk ... nearly!

I have finally got to the end of this walk. As I put pen to paper and keyboard to screen it is early November 2011. It's not hard to figure out when this started—just look at the early new moon dates investigated in this study. The first one mentioned was March 6. So here we are 8 months later. It has been a very interesting walk. An awful lot of ground has been covered in these months. Maybe you do not agree with my conclusions but I hope you have enjoyed the walk.

... and then just about 3 years on ...

Important Historical Update! [Sep 2014]

An historical search for the crucifixion date has revealed that only the waxing crescent approach was successful in landing on a Friday. 3 years were tried that should be reasonably close given Daniel's prophecy for the time of the Messiah's death: AD 30, 31, or 32. We couldn't find a Friday date using the beginning of the dark phase of the moon for the Biblical new moon worship day.

Note: we also looked at AD 33 with similar results.

This Biblical date approach is something I tried a few years ago but without success. This time it worked. And finding a reasonable date for the crucifixion basically verified just which new moon approach was most likely to be the correct new moon worship day of the Bible. The waxing crescent won. Time permitting I will adjust my pages to reflect this result.

And here's a link to this study...

Finally to finish off we add a puzzle here for our atheist friends. A quote from the Bible and a wonderful bit of information about the moon from a site that probably doesn't have any Christian leaning. Enjoy!

Gen 1:16 And God made the two great lights, the greater light to rule the day, and the lesser light to rule the night; he made the stars also.

The Moon orbits the Earth - or more accurately the Earth and Moon revolve around a common barycentre - at a centre-to-centre distance of 384,000 km. The Sun, some 150,000,000 km away, is 1,400,000 km across. It is an almost unbelievable coincidence that from the surface of the Earth, the Sun and the Moon appear more or less exactly the same size - around 32 arc minutes. It is this fact which allows us to see the Sun’s corona during a solar eclipse. No other combination of bodies anywhere in the Solar System give rise to this phenomenon.
http://www.inconstantmoon.com/not_dime.htm

Stephen Buckley

POSTSCRIPT

The above study basically ended in November of 2011. The original plan was to show here how the Jewish model shaped up with each current year. However, it did not do well for 2013 so this approach will not be followed. This is further commented on below. First, how did it do for 2012?



As you can see for 2012 the Jewish Model returned very reasonable dates. But notice what happens when we try the Jewish Model for 2013...



Only 3 of the proposed dates were in agreement with the Jewish calendar for 2013! At first I thought that the Jewish Model had completely bungled it. But the problems here were actually far beyond any numerically proposed value for the Jewish Model. It appears that the Jewish Calendar for 2013 shows just what sort of problems you can have by rigidly adhering to a fixed value for the length of the lunar synodic month as the moon wanders through the year. Have a real close look at the Jewish Rosh Chodesh dates given in the last column and note the superscripts.

It appears that for 2013 the Jewish Calendar actually drifted to the point where half of the Jewish Rosh Chodesh dates given actually fell on the same day as the Astronomical New Moon! Further than this, the Jewish Calendar had actually drifted so far that 2 of the Jewish Rosh Chodesh dates fell BEFORE the Astronomical New Moon and one of them actually fell on the same day that the NT Bible Model proposed and these were for the beginning of the Dark Moon phase!!!

Anyway this has already been commented upon in the previous 2013 insert. The Jews readily acknowledge these discrepancies.

What do these results mean for the numerical value for the Jewish Model proposed in this study? Since 2 of the Rosh Chodesh dates given in the Jewish calendar for 2013 actually fell before the Astronomical New Moon, NO numerical value will work properly with the Jewish calendar.

And what do these results mean for the NT Bible Model proposed in this study? If nothing else, strengthen it! The NT Bible Model does follow the moon correctly through the year.

There is nothing wrong with using a fixed value for the months as in the Jewish calendar. It makes more sense to have months a fixed length instead of continually wandering each year with different values. At least for calendar construction, this side of time.

However, as I have found in this study, anyone wanting to keep the Biblical New Moon worship day as mentioned in Isaiah and Revelation for the coming ages of eternity should find the NT Bible Model approach here constructed to be of some use.

Note: it is possible to use the NT Bible Model approach to work approximations for the crescent version also. And according to the historical update given above this is the direction the NT Bible Model is going to take.

EXTRA NOTES, REFERENCES etc

William Gesenius' Hebrew and English Lexicon of the Old Testament. Edited by Francis Brown, S.R.Driver, and Charles A. Briggs.

Though this study ended in November of 2011 there have been some minor amendments.

The general inserts for years [2013 etc] are pretty obvious.

April 2013 added 3 verses to the Psalm 81 section, same verse, just added the following translations:
Jewish Publication Society Bible 1917;
Complete Jewish Bible 1998; and
The Jewish Study Bible, TANAKH Translation, 1999.

10 May 2014 new background and heading.
30 Aug 2014 stating that crescent approach is more common but Bible may suggest dark phase as a possible alternative. May use NT Bible Model approach in future to also work crescent approximations similar to Jewish model but without any adjustment.
9 Sep 2014 Historical update outlining future direction of the NT Bible Model as an approximation for the waxing crescent instead of the beginning of the dark phase of the moon.

17,13 Oct 2019 new background and heading.

14 Nov 2021 fixed up typos "leeway of 8.10 − 4.36 = 3.24 or 3 hours and 24 mins" when this should have been 3.34 or 3 hours and 34 mins. The number 3.34 [3hrs 34mins] was used in the calculations so no corrections were needed there.
Added Quote Notes.

Proposal selection amendment/update for 2022 onwards
Using the numerical 1.5 day point as the beginning of the new moon day a simplified amended proposal follows:
From sunset onwards select the following day since in theory the days are kept from sunset to sunset. If the numerical value falls before sunset also use that day.
Using this approach sunset time checks would only be needed in these curious afternoon decisions.
Also a more laid back approach would be to ignore the following day if the end of the numerical day is in the early morning during the dark part of the day.
See Quote Note 1.

Show/Hide Quote Notes text etc

1.
The NASA page for this appears to be long gone. The following quote gives some similar information:

According to NASA, a full moon technically only lasts for the moment when the Earth is directly between the sun and the moon. However, full moons appear to last for approximately three days.
Over the course of approximately 28 days, the moon goes through different phases based on the relative positions of the Earth, the moon and the sun. A true full moon only occurs during the instant when the moon appears completely illuminated to observers on Earth. However, when most people talk about the full moon, they are referring to the several-day period when the moon appears to be at least 95 percent full.
https://www.reference.com/science/many-days-full-moon-last-588a1e5f7d59ab60

Embarrassing afterthought:
This one quote gives the numerical solution to my model in one swift hit. Half of three days, or 1.5 days. That's it!
How do I arrive at this? Remember that the mathematics gave the astronomical new moon [dark moon] and full moon phases as the longest. And they would be equal. So by this quote the astronomical new moon [dark moon] theoretically should last visibly for approximately three days. That's it.
Mathematical reasoning: If the Jewish "new moon" is recognized from the first visible sliver of the waxing crescent then this must not occur until the astronomical new moon [dark moon] phase has visibly ended. If the astronomical new moon [dark moon] phase is approximately 3 days long then that is from 1.5 days before the conjuction to 1.5 days after it. So at 1.5 days after the conjunction we can assume numerically that the Jewish new moon phase should start. That's Rosh Chodesh.
This also led to the
Proposal selection amendment/update for 2022 onwards section. See above.

2.
Information now at:
https://www.jewishvirtuallibrary.org/rosh-chodesh

3.
The NASA page for this appears to be long gone.
However, nearly identical information can be found at:
http://astropixels.com/ephemeris/phasescat/phases2001.html

4.
Regardless of the culture, all lunar calendar months approximate the mean length of the synodic month, the average period the Moon takes to cycle through its phases (new, first quarter, full, last quarter) and back again: 29–30 days.
The average duration in modern times is 29.53059 days
http://en.wikipedia.org/wiki/Lunar_month

5.
The period of the Moon's orbit as defined with respect to the celestial sphere of apparently fixed stars is known as a sidereal month because it is the time it takes the Moon to return to a similar position among the stars (Latin: sidera): 27.321661 days (27 d 7 h 43 min 11.6 s).
http://en.wikipedia.org/wiki/Lunar_month

6.
While the Moon is orbiting the Earth, the Earth is progressing in its orbit around the Sun. After completing a sidereal month, the Moon must move a little further to reach the new position having the same angular distance from the Sun, appearing to move with respect to the stars since the previous month. Therefore, the synodic month takes 2.2 days longer than the sidereal month.
http://en.wikipedia.org/wiki/Lunar_month

7.
The eccentricity of Earth's orbit is currently about 0.0167; its orbit is nearly circular.
http://en.wikipedia.org/wiki/Orbital_eccentricity

8.
In mathematics, an ellipse is a plane curve surrounding two focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. As such, it generalizes a circle, which is the special type of ellipse in which the two focal points are the same. The elongation of an ellipse is measured by its eccentricity e, a number ranging from e = 0 (the limiting case of a circle) to e = 1 (the limiting case of infinite elongation, no longer an ellipse but a parabola).
http://en.wikipedia.org/wiki/Ellipse

9.
Page long gone but info can now be found at:
http://www.hillel.org/docs/default-source/mcms-file-archives/rosh_chodesh_groups_guide.pdf?sfvrsn=2
which opens a pdf doc:
Hillel's Joseph Meyerhoff Center for Jewish Learning
Rosh Chodesh Groups Guidebook
The title and paragraph start on page 6.

10.

Rosh Chodesh
Rosh Chodesh 101
A minor holiday that marks the beginning of each Hebrew month.
Rosh Chodesh — which literally means "head of the month" – is the minor holiday that marks the beginning of every Hebrew month.
https://www.myjewishlearning.com/article/rosh-chodesh-101/

11.
Now at:
https://en.wikipedia.org/wiki/Hebrew_calendar#Determining_the_new_month_in_the_Mishnaic_period

12.
Page long gone but same info looks to be at:
https://etzion.org.il/en/talmud/seder-moed/massekhet-rosh-hashana/kiddush-ha-chodesh-and-birkat-ha-chodesh

13.
The Jewish Calendar info can now be found at:
https://bje.org.au/knowledge-centre/jewish-calendar/

14.
Link problems. But a similar/identical version of this map can be found at:
https://webarchive.library.unt.edu/web/20130213210928/http://aa.usno.navy.mil/faq/docs/world_tzones.php

15.
I have checked the oldest version of this page and no link url was given for this. But I did find the related doc dating to about 2007 which I had downloaded possibly about March 2011 while I was putting this study together. My initial page construction is given about April 2011 so it fits.
A current link gives this info in a pdf doc:
https://gatherthepeople.org/Downloads/ROSH_CHODESH.pdf

16.
A month is a unit of time, used with calendars, that is approximately as long as a natural orbital period of the Moon; the words month and Moon are cognates. The traditional concept arose with the cycle of Moon phases; such lunar months ("lunations") are synodic months and last approximately 29.53 days.
https://en.wikipedia.org/wiki/Month

17.
In linguistics, cognates, also called lexical cognates, are words that have a common etymological origin.
The word cognate derives from the Latin noun cognatus, which means "blood relative".
https://en.wikipedia.org/wiki/Cognate

18.
This NASA quote/page is long gone, but the nearly identical information can be found at:

the mean synodic month (New Moon to New Moon)
http://astropixels.com/ephemeris/phasescat/phases2001.html

19.
Also called lu·nar month . the period of a complete revolution of the moon around the earth, as the period between successive new moons (synodic month ), equal to 29.531 days, or the period between successive conjunctions with a star (sidereal month ), equal to 27.322 days, or the period between successive perigees (anomalistic month ), equal to 27.555 days, or the period between successive similar nodes (nodical month, or draconic month ), equal to 27.212 days.
https://www.dictionary.com/browse/month

20.
This page looks long gone. Similar info follows:

Merism (Latin merismus, Greek μερισμός merismós) is a rhetorical device (or figure of speech) in which a combination of two contrasting parts of the whole refer to the whole.

Merisms are common in the Old Testament. For example, in Genesis 1:1, when God creates את השמים ואת הארץ (Modern pronunciation: et hashamaim ve-et haarets) "the heavens and the earth" (New Revised Standard Version), the two parts (heavens and earth) do not refer only to the heavens and the earth. Rather, they refer to the heavens, the earth and everything between them, i.e. God created the entire world, the whole universe. Other famous examples of Biblical merisms are Genesis 1:5, where "evening" and "morning" refer to "one day" (including noon, afternoon etc.); and Psalm 139, where the psalmist declares that God knows "my downsitting and my uprising", i.e. God knows all the psalmist's actions.
https://en.wikipedia.org/wiki/Merism

21.
(literature, rhetoric) A reference to something by its polar extremes, as in "we searched high and low".
https://en.wiktionary.org/wiki/merism

22.
the Moon does not orbit the exact center of the Earth, but a point on a line between the center of the Earth and the Moon, approximately 1,710 km (1,062 miles) below the surface of the Earth, where their respective masses balance. This is the point about which the Earth and Moon orbit as they travel around the Sun.
http://en.wikipedia.org/wiki/Center_of_mass#Astronomy

23.
Page long gone but info can be found at:
https://faculty.kfupm.edu.sa/PHYS/alshukri/astro/crest2.htm
Note:
The title "Crescent visibility" is here part of the main title of the whole article.
This info is nearly identical but has the telescope time replaced with binoculars.
The naked-eye record is also changed to 15.4 hours from 15.5.

24.
Some of these links work. A couple of them look dead. And one works but can't do the year required.

25.
The chabad.org page appears to only give current values. The molad times for 2011 can be found at:
https://torahcalc.com/molad/

26.
A synodic month is the most familiar lunar cycle, defined as the time interval between two consecutive occurrences of a particular phase (such as new moon or full moon) as seen by an observer on Earth. The mean length of the synodic month is 29.53059 days (29 days, 12 hours, 44 minutes, 2.8 seconds). Due to the eccentricity of the lunar orbit around Earth (and to a lesser degree, the Earth's elliptical orbit around the Sun), the length of a synodic month can vary by up to seven hours.
https://en.wikipedia.org/wiki/Month#Synodic_month
I question this. For 2011 I found the range to be about 10 hours. Further, note the following quote:

Length of Synodic Month: 2001 to 2100
During the 100-year period covered in this catalog, there are 1236 complete lunations. The shortest lunation begins on 2053 Jun 16 at 10:51 GMT and lasts 29d 06h35m (06h 09m shorter than the mean). The longest lunation begins on 2017 Dec 18 at 06:31 GMT and lasts 29d 19h47m (07h 03m longer than the mean). Thus, the duration of the lunation varies over a range of 13h 12m during this century.
http://astropixels.com/ephemeris/moon/synodicmonth2001.html

Well that puts it to rest. For this century, 13 hours and 12 mins.
An afterthought, they may have meant vary from the mean length, or very roughly 7 hours max. It's very close and it would explain the quote.
Hide text

1.
The NASA page for this appears to be long gone. The following quote gives some similar information:

According to NASA, a full moon technically only lasts for the moment when the Earth is directly between the sun and the moon. However, full moons appear to last for approximately three days.
Over the course of approximately 28 days, the moon goes through different phases based on the relative positions of the Earth, the moon and the sun. A true full moon only occurs during the instant when the moon appears completely illuminated to observers on Earth. However, when most people talk about the full moon, they are referring to the several-day period when the moon appears to be at least 95 percent full.
https://www.reference.com/science/many-days-full-moon-last-588a1e5f7d59ab60

Embarrassing afterthought:
This one quote gives the numerical solution to my model in one swift hit. Half of three days, or 1.5 days. That's it!
How do I arrive at this? Remember that the mathematics gave the astronomical new moon [dark moon] and full moon phases as the longest. And they would be equal. So by this quote the astronomical new moon [dark moon] theoretically should last visibly for approximately three days. That's it.
Mathematical reasoning: If the Jewish "new moon" is recognized from the first visible sliver of the waxing crescent then this must not occur until the astronomical new moon [dark moon] phase has visibly ended. If the astronomical new moon [dark moon] phase is approximately 3 days long then that is from 1.5 days before the conjuction to 1.5 days after it. So at 1.5 days after the conjunction we can assume numerically that the Jewish new moon phase should start. That's Rosh Chodesh.
This also led to the
Proposal selection amendment/update for 2022 onwards section. See above.

2.
Information now at:
https://www.jewishvirtuallibrary.org/rosh-chodesh

3.
The NASA page for this appears to be long gone.
However, nearly identical information can be found at:
http://astropixels.com/ephemeris/phasescat/phases2001.html

4.
Regardless of the culture, all lunar calendar months approximate the mean length of the synodic month, the average period the Moon takes to cycle through its phases (new, first quarter, full, last quarter) and back again: 29–30 days.
The average duration in modern times is 29.53059 days
http://en.wikipedia.org/wiki/Lunar_month

5.
The period of the Moon's orbit as defined with respect to the celestial sphere of apparently fixed stars is known as a sidereal month because it is the time it takes the Moon to return to a similar position among the stars (Latin: sidera): 27.321661 days (27 d 7 h 43 min 11.6 s).
http://en.wikipedia.org/wiki/Lunar_month

6.
While the Moon is orbiting the Earth, the Earth is progressing in its orbit around the Sun. After completing a sidereal month, the Moon must move a little further to reach the new position having the same angular distance from the Sun, appearing to move with respect to the stars since the previous month. Therefore, the synodic month takes 2.2 days longer than the sidereal month.
http://en.wikipedia.org/wiki/Lunar_month

7.
The eccentricity of Earth's orbit is currently about 0.0167; its orbit is nearly circular.
http://en.wikipedia.org/wiki/Orbital_eccentricity

8.
In mathematics, an ellipse is a plane curve surrounding two focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. As such, it generalizes a circle, which is the special type of ellipse in which the two focal points are the same. The elongation of an ellipse is measured by its eccentricity e, a number ranging from e = 0 (the limiting case of a circle) to e = 1 (the limiting case of infinite elongation, no longer an ellipse but a parabola).
http://en.wikipedia.org/wiki/Ellipse

9.
Page long gone but info can now be found at:
http://www.hillel.org/docs/default-source/mcms-file-archives/rosh_chodesh_groups_guide.pdf?sfvrsn=2
which opens a pdf doc:
Hillel's Joseph Meyerhoff Center for Jewish Learning
Rosh Chodesh Groups Guidebook
The title and paragraph start on page 6.

10.

Rosh Chodesh
Rosh Chodesh 101
A minor holiday that marks the beginning of each Hebrew month.
Rosh Chodesh — which literally means "head of the month" – is the minor holiday that marks the beginning of every Hebrew month.
https://www.myjewishlearning.com/article/rosh-chodesh-101/

11.
Now at:
https://en.wikipedia.org/wiki/Hebrew_calendar#Determining_the_new_month_in_the_Mishnaic_period

12.
Page long gone but same info looks to be at:
https://etzion.org.il/en/talmud/seder-moed/massekhet-rosh-hashana/kiddush-ha-chodesh-and-birkat-ha-chodesh

13.
The Jewish Calendar info can now be found at:
https://bje.org.au/knowledge-centre/jewish-calendar/

14.
Link problems. But a similar/identical version of this map can be found at:
https://webarchive.library.unt.edu/web/20130213210928/http://aa.usno.navy.mil/faq/docs/world_tzones.php

15.
I have checked the oldest version of this page and no link url was given for this. But I did find the related doc dating to about 2007 which I had downloaded possibly about March 2011 while I was putting this study together. My initial page construction is given about April 2011 so it fits.
A current link gives this info in a pdf doc:
https://gatherthepeople.org/Downloads/ROSH_CHODESH.pdf

16.
A month is a unit of time, used with calendars, that is approximately as long as a natural orbital period of the Moon; the words month and Moon are cognates. The traditional concept arose with the cycle of Moon phases; such lunar months ("lunations") are synodic months and last approximately 29.53 days.
https://en.wikipedia.org/wiki/Month

17.
In linguistics, cognates, also called lexical cognates, are words that have a common etymological origin.
The word cognate derives from the Latin noun cognatus, which means "blood relative".
https://en.wikipedia.org/wiki/Cognate

18.
This NASA quote/page is long gone, but the nearly identical information can be found at:

the mean synodic month (New Moon to New Moon)
http://astropixels.com/ephemeris/phasescat/phases2001.html

19.
Also called lu·nar month . the period of a complete revolution of the moon around the earth, as the period between successive new moons (synodic month ), equal to 29.531 days, or the period between successive conjunctions with a star (sidereal month ), equal to 27.322 days, or the period between successive perigees (anomalistic month ), equal to 27.555 days, or the period between successive similar nodes (nodical month, or draconic month ), equal to 27.212 days.
https://www.dictionary.com/browse/month

20.
This page looks long gone. Similar info follows:

Merism (Latin merismus, Greek μερισμός merismós) is a rhetorical device (or figure of speech) in which a combination of two contrasting parts of the whole refer to the whole.

Merisms are common in the Old Testament. For example, in Genesis 1:1, when God creates את השמים ואת הארץ (Modern pronunciation: et hashamaim ve-et haarets) "the heavens and the earth" (New Revised Standard Version), the two parts (heavens and earth) do not refer only to the heavens and the earth. Rather, they refer to the heavens, the earth and everything between them, i.e. God created the entire world, the whole universe. Other famous examples of Biblical merisms are Genesis 1:5, where "evening" and "morning" refer to "one day" (including noon, afternoon etc.); and Psalm 139, where the psalmist declares that God knows "my downsitting and my uprising", i.e. God knows all the psalmist's actions.
https://en.wikipedia.org/wiki/Merism

21.
(literature, rhetoric) A reference to something by its polar extremes, as in "we searched high and low".
https://en.wiktionary.org/wiki/merism

22.
the Moon does not orbit the exact center of the Earth, but a point on a line between the center of the Earth and the Moon, approximately 1,710 km (1,062 miles) below the surface of the Earth, where their respective masses balance. This is the point about which the Earth and Moon orbit as they travel around the Sun.
http://en.wikipedia.org/wiki/Center_of_mass#Astronomy

23.
Page long gone but info can be found at:
https://faculty.kfupm.edu.sa/PHYS/alshukri/astro/crest2.htm
Note:
The title "Crescent visibility" is here part of the main title of the whole article.
This info is nearly identical but has the telescope time replaced with binoculars.
The naked-eye record is also changed to 15.4 hours from 15.5.

24.
Some of these links work. A couple of them look dead. And one works but can't do the year required.

25.
The chabad.org page appears to only give current values. The molad times for 2011 can be found at:
https://torahcalc.com/molad/

26.
A synodic month is the most familiar lunar cycle, defined as the time interval between two consecutive occurrences of a particular phase (such as new moon or full moon) as seen by an observer on Earth. The mean length of the synodic month is 29.53059 days (29 days, 12 hours, 44 minutes, 2.8 seconds). Due to the eccentricity of the lunar orbit around Earth (and to a lesser degree, the Earth's elliptical orbit around the Sun), the length of a synodic month can vary by up to seven hours.
https://en.wikipedia.org/wiki/Month#Synodic_month
I question this. For 2011 I found the range to be about 10 hours. Further, note the following quote:

Length of Synodic Month: 2001 to 2100
During the 100-year period covered in this catalog, there are 1236 complete lunations. The shortest lunation begins on 2053 Jun 16 at 10:51 GMT and lasts 29d 06h35m (06h 09m shorter than the mean). The longest lunation begins on 2017 Dec 18 at 06:31 GMT and lasts 29d 19h47m (07h 03m longer than the mean). Thus, the duration of the lunation varies over a range of 13h 12m during this century.
http://astropixels.com/ephemeris/moon/synodicmonth2001.html

Well that puts it to rest. For this century, 13 hours and 12 mins.
An afterthought, they may have meant vary from the mean length, or very roughly 7 hours max. It's very close and it would explain the quote.

Page design/construction 2011.